Consider the motion of an airplane in flight. A time-varying vector describes the velocity (km/h) of the passengers as the plane travels between two cities. At a particular time, the magnitude of the velocity vector gives the passengers' speed without regard to the heading. The three directional components (x, y, z) of the velocity vector at some time will give the speed with respect to its position in space, described by a position vector in altitude, longitude, and latitude coordinates. Over time, a variety of measures could be chosen to summarize the passengers' time-varying velocity vector. For example, the average vector magnitude would describe their average speed, or the average vertical position would give their average altitude. We could even choose some measure of change in the vector components with time to describe features such as the rate of descent or acceleration. All of these summary measures are equally valid in a physical sense but describe qualitatively different aspects of the passengers' motion that may not be equally important in relation to a biological response.
The challenge for exposure assessment is to choose a summary measure that is physically meaningful and biologically relevant. To illustrate this point, the average speed of the passengers may be useful for deciding how long their trip will take but is essentially useless for predicting whether the passengers will become airsick; however, another summary measure, describing velocity fluctuations up and down, would probably be a very good predictor of possible air sickness during a flight. A different summary measure, involving the directional components of the motion, would be needed to predict another biological response such as jet lag. Thus, the choice of summary measure depends on both the physical characteristic to be described and the biological response of interest.
An electromagnetic field is composed of two components, the electric and the magnetic fields. The electric field is created by the presence of an electric charge. It describes the magnitude and direction of the force it exerts on a positive electric charge. The magnitude of the electric field depends on the difference in potential between charge-carrying bodies, including conductors, regardless of the amount of current that is flowing in them. In contrast, a magnetic field is created by the motion of electric charges. Typically, this motion is represented by a flow of charge in the form of an electric current, which gives the number of charges per second passing through the conductor. The magnetic field acts only on other electric charges in motion. Thus, a magnetic field is created by an electric current and describes the magnitude and direction of the force exerted on a nearby current (moving charges). The magnitude of the magnetic field is proportional to the current flow in a conductor, regardless of the voltage present.
The discussion in this document focuses primarily on magnetic fields but will include electric fields when possible because of their inherently close association with electric power systems. Voltage and current determine the magnitude of the electric and the magnetic fields at a location, respectively, with the source geometry and distance from the source to the measurement location. The strength of an electric field is usually measured in volts per meter (V/m) or sometimes in kilovolts per meter (1 kV/m = 1000 V/m). Magnetic fields can be designated by either magnetic flux density (B) or magnetic field strength (H); both are proportional to the magnitude of the current. B is measured in the centimeter-gram-second unit, the gauss (G), or the unit of the Systeme Internationale (SI), the tesla (T); 1 mG = 1 x 10-3 G = 0.1 T. H is measured in SI units of amperes/meter (A/m). B and H are related through the equation: B = µ0H, where µ0 = 1.26 x 10-6 henry/m is the magnetic permeability of a vacuum. To a close approximation, µ0 remains the same for air and body tissues, and only one of the variables, B or H, need be measured. In practice, B is the usual measured quantity, and, for the purpose of this document, 'magnetic field' refers to the magnetic flux density in microtesla (T; 1 T = 1 x 10-6 T). Unless otherwise stated, all voltage, current, and magnetic flux values are rms (root mean square), as defined in equation 2.4.
EMF can be arranged in an orderly fashion in an electromagnetic spectrum, according to their frequency (f) or wavelength (), where = c/f and c is the velocity of light. The electromagnetic spectrum spans an enormous range of frequencies (Figure 2.1), more than 15 orders of magnitude. This document focuses principally on EMF resulting from the use and distribution of electric power, allowing a great deal of simplification as these fields vary rather slowly over time. The frequency of EMF depends on the power-line source; in North America, power systems operate at a frequency of 60 cycles per second, or 60 hertz (Hz). Generally, these power-line fields fall in the extremely low frequency (ELF) region of the electromagnetic spectrum, which is defined by frequencies from 3 to 3000 Hz (Poole & Ozonoff, 1996). The alternating current flowing in the electric power system has dominant sinusoidal voltage and current waveforms; however, although 60 Hz is the predominant fundamental frequency, humans are exposed to a mixture of frequencies, and much higher frequencies can arise. For example, switching events can generate abrupt spikes in voltage and current waveforms, leading to high frequency 'transients', which can extend into radio frequencies above several megahertz (1 MHz = 106 Hz). Nonlinear characteristics in electrical devices can generate harmonics at integer multiples of the fundamental frequency extending up to several kilohertz (1 kHz = 103 Hz). EMF frequencies from some electronic equipment, like televisions and visual display terminals (VDT), can routinely extend up to 50 kHz.
Most devices that have electric wires, e.g. electric motors, electric equipment such as electric power lines, residential appliances, and industrial equipment, are potential sources of EMF. Residential exposures are dominated by ELF sources but also include exposure to very low frequencies, 3-30-kHz radio frequencies, and microwave sources.
The Earth also produces EMF. Unlike the fields from power lines and other alternating current sources, the Earth's fields are largely 'static', that is they do not change over time. In contrast, EMF from power lines and other alternating current sources have a periodic component. The Earth's magnetic field has a magnitude of about 50 T over most of the USA and is oriented toward magnetic North; the vertical field component accounts for about two-thirds of the total vector magnitude. Devices that operate on direct current (DC) also produce static magnetic fields, and some occupational environments, such as aluminum smelting, can have extremely strong static fields.
For the most part, researchers have focused on ELF magnetic fields resulting from power lines, appliances, and occupational exposures. In the broader context of human exposures and epidemiological studies, it should be remembered that typical exposures to EMF occur over a wide range of frequencies and in conjunction with static fields.
ELF electric fields at relatively high intensities can have acute biological effects. Nerve and muscle stimulation results in an immediate behavioral response in humans and other vertebrates (Malmivuo & Plonsey, 1995; Reilly, 1992). Extremely strong electric fields can permanently or transiently damage cell membranes (Weaver & Chizmadzhev, 1996) and produce burn injuries (Tropea & Lee, 1992). Stimulation of peripheral nerves at power frequencies in humans generally requires electric current densities in muscle tissue in the order of 1.0 A/m2, which corresponds roughly inside tissues with an electric conductivity of s 1 S/m to internal electric fields on the order of 1.0 V/m. Production of such currents inside living tissue at 60 Hz requires either direct, electrically conductive contact with a source of electric power or the presence of an electric field in the surrounding air in the order of several 100 kV/m. Without conductive contact, the electric field outside body tissues (EOUT) must be much larger than the field inside (EINS). This requirement is a consequence of the physical laws of conservation of electric charge and continuity of electric displacement. In the case of steady-state sinusoidal electric fields of frequency f at a plane boundary between 'semi-infinite' media, the ratio of the external electric field over the internal field is described by the relation (Polk, 1986):
![]() Eq. 2.1
Eq. 2.1
where f, is the dielectric permittivity of air and s is the conductivity of tissue 1 S/m. Since for dry air 8.84 x 10-12 F/m, the ratio given by equation 2.1, which describes the attenuation of an external electric field relative to the apparent field inside the body, is roughly 100 million. Distortion of the field in air by the presence of a 'conducting' body, air moisture, contact with an electrically conducting earth, and variation of s within the body, can reduce this ratio somewhat, but it will never be much less than 10 million. Details of current and field distributions that take into account body orientation and in homogeneity have been given, for example, by Kaune (Kaune & Forsythe, 1985) and Dawson (Dawson et al., 1996).
Typical 60 Hz electric fields in homes rarely exceed 100 V/m (Barnes et al., 1989) and are not more than 10 kV/m near ground level directly underneath a very high-voltage (~ 500 kV) transmission line. It is clear that the only persons who will experience internal electric fields in the order of even 10-3 V/m due to an external electric field are utility workers in the immediate vicinity of high-voltage wires.
Time-varying magnetic fields induce electric fields into any material, according to Faraday's law:
![]() Eq. 2.2
Eq. 2.2
The term on the left-hand side of the equation indicates the electric
field integrated over a closed boundary; the term on the right-hand side
is the integral of the rate of change of the magnetic flux density, B,
perpendicular to and integrated over the area enclosed by the contour designated
on the left-hand side . The integral ![]() is also called the magnetic flux f. For a reasonably
long, cylindrical body of radius r, eq. 2.2 leads to (Polk, 1986):
is also called the magnetic flux f. For a reasonably
long, cylindrical body of radius r, eq. 2.2 leads to (Polk, 1986):
E = p f B r Eq. 2.3
where B is the magnetic flux in tesla and r is in meters, which is frequently used to estimate the average electric field induced by a time-varying magnetic field. Since the left-hand side of equation 2.2 gives only the induced electric field summed over a closed contour, equation 2.3 applies only to an electrically homogeneous medium; however, in electrically inhomogeneous tissue, this induced field can vary locally, giving rise to much larger or smaller values than the average (Polk, 1992c).
The magnetic permeability (µ) of living tissue (with very few, localized exceptions) is practically equal to that of free space. Consequently, the magnetic flux density inside the body is nearly equal to that outside. Equation 2.3 then indicates that a 60 Hz field of 100 µT oriented along the head-to-feet axis of a human, with an average radius of 15 cm, will induce near the periphery of the body an average electric field of 2.8 x 10-3 V/m. In comparison, the average electric field near the rim of a petri dish, with a radius of 3 cm, induced by a vertical 60 Hz, 100-µT magnetic field will be about 0.56 x 10-3 V/m.
These magnetically induced internal electric fields are very much larger than those due to a 100-V/m external electric field in air. A 100-V/m electric field represents the upper limit of those found in typical homes; however, induced electric fields are still much smaller than internal electric fields associated with nerve and muscle stimulation. The average electric field induced by a 0.3-µT 60 Hz field near the surface of a human with a 15-cm radius is only 8.5 x 10-6 V/m. Thus, typical magnetic fields encountered in epidemiological studies of residences induce internal currents and electric fields that are roughly one million times smaller than the currents required to produce acute nerve and muscle stimulation.
The basic laws derived from 'classical' physics, which can operate either directly or indirectly and at either the macroscopic or the microscopic (molecular) level in field-biosystems interactions, are summarized in Figures 2.2 and 2.3; quantum mechanical considerations are discussed in Section 4.7.5. Forces and torques are given for both electric fields and magnetic flux densities. Individual electric charges, electric dipoles, and magnetic dipoles or magnetic moments are considered. These equations indicate that stationary or moving individual electric charges will undergo translatory motion in the direction of an applied electric field. In comparison, only charges that are already moving at some velocity, v, will be subjected to a magnetic or 'Lorentz' force in a magnetic flux density, B, that is perpendicular to both v and B (as indicated by the cross-product). This perpendicular force on electric charges in a magnetic field leads to a circular motion and the 'cyclotron resonance' phenomenon (Liboff, 1985), which will appear only when the probability of collisions with stationary or randomly moving particles is extremely small (Durney et al., 1988). Translatory motion of electric dipoles, 'dielectrophoresis' (Pohl, 1978), and of magnetic moments requires very large spatial field gradients (Barnes, 1986). The appearance of torques, producing rotation, requires either the pre-existence of dipole moments or their generation by an applied field. This includes phenomena at the atomic level, where the nuclear magnetic moment and nuclear angular momentum are responsible for nuclear magnetic resonance at frequencies determined by an applied DC magnetic field.
Various biophysical mechanisms have been proposed to account for the effects of EMF (see section 4.8). Some mechanisms predict a strong (or weak) dependence on the frequency content, magnitude, or spatial direction of the fields. Some mechanisms proposed for the interaction of magnetic field with tissues suggest a response from both the time-varying and static-field components or specify a unique combination of the static and time-varying field vectors for a particular target molecule. Also, we cannot ignore the possible role of the state of the biological system, for example awake or sleeping, which could make the timing of exposure important. A great variety of possible exposure factors could be considered, none of which can be excluded a priori, and several different mechanisms have been postulated. Assumptions must thus be made when measuring EMF and constructing a few simple summary measures of exposure for the purposes of health assessment.
![]() Eq. 2.4
Eq. 2.4
where B(t) is the instantaneous magnetic flux density and T is the time for an integral over a number of periods of the fundamental frequency.
For a pure sinusoidal waveform, the rms value is related to the instantaneous peak value by a factor of 2, i.e. Bpeak = 2 Brms. Similar formulas apply for computing the rms voltage. The rms value is always positive and can be related to the average power in watts (energy per unit time), where watts are defined by the instantaneous product of voltage and current delivered to a load. Note that ultimately it is the integral of power with time (i.e. watt-hours or kilowatt-hours) that represents the amount of energy delivered by a utility to a customer.
The measurements of EMF used in most studies are records of the magnitude of the field; they do not retain information on the directional orientation in space or changes in direction over time. This is accomplished by measuring the rms value of three orthogonal spatial components (x, y, and z), and then combining these three values to find the magnitude of the rms. This value, sometimes referred to as the rms resultant, is computed as:
![]() Eq. 2.5
Eq. 2.5
where Bx , By, and Bz are the orthogonal magnetic flux density spatial components. Throughout this document, the quantity Bres is referred to as the magnitude of the magnetic field or vector. As noted above, the magnitude does not depend on the direction of the field vector in space. Therefore, Bres provides an isotropic measure that does not depend on the orientation of the coordinate system for the measuring device. This is a convenient property for personal exposure meters, in which the spatial orientation constantly changes as the subject moves about.
In many studies, instruments have been used to measure or estimate the time-weighted average (TWA) magnitude of the magnetic field. The TWA is computed from the equation:
![]() Eq. 2.6
Eq. 2.6
where T is the averaging time.
Note that for a series of measurements uniformly spaced over time, the TWA reduces to the simple average (mean) of the values over T, the time interval. The TWA is by far the commonest exposure metric. It represents a measure of field magnitude, averaged over time. These definitions have been given in terms of magnetic field components, but similar quantities can be derived for electric fields.
The electric power system in North America is arranged in a series of building blocks or segments, with power lines connecting generating stations via a network of transmission lines, intermediate substations, and switching points to the local distribution lines, and ultimately to utility customers. By design, the voltage in a given portion of the system remains nearly constant; large transmission lines typically operate at > 35 kV, and primary distribution lines operate at about 4-35 kV. These higher voltages on the transmission lines are stepped down at local substations by transformers to produce the voltages for the primary distribution lines. The primary distribution lines are further reduced by transformers at other points to a correspondingly lower voltage and higher current for residential or commercial use. Typically, residential service operates at 120 and 240 V. Roughly speaking, the power delivery in each segment of the electric system is the product of the voltage and current load. So power is delivered by creating high voltage at moderate current in the transmission segments and transforming this into high current at moderate voltage for residential distribution. An important consequence of this distribution system is that transmission lines are usually larger sources of electric fields, because of the high line voltage. In most cases, transmission lines carry larger load currents than primary distribution lines but are located farther away from residences; however, in some cases transmission lines carry load currents similar to those of primary distribution lines. Thus, overall, commercial and residential power distribution systems can be a more significant source of magnetic fields than transmission lines but are usually not a very significant source of large electric fields.
Although the voltage in a given segment of the power system remains nearly constant, the current in each portion is highly variable over time, depending on the changing demand for electric power. As a result, in the absence of other changes (such as introducing shielding materials) at locations near power lines, electric fields remain fairly constant, while magnetic fields generally vary significantly over time. Interestingly, residential measurements of magnetic fields show appreciable morning and evening peaks (Dovan et al., 1993) and a seasonal component which varies by geographic region and closely follows the electrical use patterns of urban residents. Consequently, the timing of measurements during a day or season can lead to systematic bias in estimates of 24-h or annual average exposures (Bracken et al., 1993).
Calculations of EMF frequently involve a spatial arrangement of one or more charge or current elements, known as multipoles (Lorrain & Corson, 1970). A monopole consists of a single positive or negative electric point charge; a small displacement of this point charge and replacement of the original charge element by a charge of equal magnitude but opposite sign creates an electric dipole. A magnetic dipole represents the basic element that describes the magnetic field from a current loop. A small displacement of a dipole and replacement of the original by a dipole of equal magnitude and opposite sign creates a quadrupole. This concept can be extended indefinitely to describe the spatial extent of fields in terms of a general multipole expansion of order 2n, where n is the number of poles. The magnetic field produced at a distance R by a multipole of order n can be described by the equation:
![]() Eq. 2.7
Eq. 2.7
with field in µT, distance in meters, and multipole in Amn, where M is the multipole vector term describing the source current. At a given frequency, the magnitude and direction of M are a function of the source current magnitude and geometry, f is the angle between the direction of M and R, and f(f) designates a function of f. Both M and B can be elliptically polarized, that is the vectors rotate in space, describing an ellipse over every cycle of the power frequency. As the distance R increases, the field produced by higher-order multipoles becomes less important. At most practical distances for exposure assessment, significant fields are produced only by terms associated with monopoles and dipoles, with order n = 1 or 2 for power lines and up to order n = 3 for appliances, with which people are nearly in contact when exposed. Magnetic monopoles do not actually exist but are a useful approximation near one pole of a large dipole.
A consequence of this dependence on geometry is that the magnitude of the magnetic field decreases fairly rapidly with movement away from an isolated source; the magnitude falls at least inversely with distance and often with the square of distance or more. A worker standing next to a magnetic field source such as a small motor might experience a field magnitude of 100 T, but by moving a few feet away the magnitude may fall to background levels of < 0.1 T. Naturally, the decrease in field strength with distance depends on the magnetic field source, and the pattern is different for a small motor and for a power line. A small motor or computer monitor is essentially a point source, in that the fields originate from a small defined area. In contrast, a power line is a line source which may decrease in strength much more slowly with distance and contribute exposure over a much larger area. Field strength at the source is only one consideration in assessing the contribution of a source to exposure to magnetic field; equally important is the individual's proximity to the source and the amount of time spent near it. Together, these factors determine the relative contribution of a source to the individual's total exposure to magnetic fields.
Exposure to EMF is assessed for many purposes, including compliance with recommended standards or regulatory limits, to evaluate the prevalence of EMF in some environment, or for use in epidemiological studies of possible health effects. The underlying purpose of the monitoring often affects the kind of information gathered, the choice of instrumentation, and the quality of the survey.
Data collected for a specific purpose may not be useful in another context. For example, data collected at a workplace in a non-random fashion or specifically to identify a worst-case exposure scenario for compliance with a standard may not provide any meaningful information on typical exposures in those jobs. The study design also must allow a sufficient sample size to achieve the desired level of precision. Another consideration is the use of assessment procedures that have been used in previous studies in order to obtain comparable results. If other data are available from the literature and consistent collection methods are used, it may be possible to combine data on exposure from several sources so as to draw broader conclusions.
As noted earlier, describing EMF with a summary measure involves many assumptions. All instruments selectively measure certain aspects of field, usually ignoring others. Consequently, no single instrument or study captures all aspects of potential human exposure to EMF. Without guidance from studies of the biomechanisms of EMF, the instrument makers made the following implicit assumptions (Heroux, 1991); (Bracken et al., 1993) :
Personal exposure monitoring was made possible by the availability of reliable compact instruments, notably the AMEX-3D, EMDEX-C, EMDEX II, EMDEX Lite, and Positron meters. All of these meters measure the rms magnitude of the magnetic field, thus sacrificing most of the information about the field's frequency content, spatial orientation, and polarization. Magnetic fields are easier to measure for personal exposure than electric fields because they are not perturbed by the body and other conductive objects; however, some meters, like the Positron and the EMDEX-C, include both electric and magnetic field sensors and provide some indication of both components. Table 2.1 summarizes the characteristics of various common exposure meters.
The EMDEX II (Electric and Magnetic Digital Exposure meter), like many other data-logging meters, measures the near 60 Hz rms directional components of the magnetic field, has some ability to distinguish higher-frequency harmonics, and can be used to monitor electric fields when fitted with a sensor. The EMDEX II covers a frequency range of about 40-800 Hz. Sahl et al. argued that these frequencies probably contain information about the biologically relevant characteristics of EMF, but biological support for that assertion is limited (Sahl et al., 1994). The simplest meter, the AMEX-3D (Average Magnetic Exposure), measures only the average magnetic field (i.e. the TWA) over time (Kaune et al., 1992). All of the personal exposure meters except the AMEX-3D provide data logging to capture temporal variation in exposures. Data loggers store measurements at regular intervals, ranging from 1 s to 1 min. Investigators may explore various exposure metrics in addition to the TWA, such as the geometric mean (GM) and the fraction of measurements that exceed a threshold. In addition to personal exposure meters, many hand-held survey meters are available for taking single spot readings of magnetic fields. A comparison of many magnetic field meters showed that most are reasonably accurate when properly calibrated (Misakian et al., 1991; Olsen et al., 1991).
EMF monitors typically have electronic filters to restrict measurement to bandwidths in the ELF range. Most instruments have inductive coil sensors which cannot measure static magnetic fields and which can produce a low-frequency signal (around 3-30 Hz), created by motion through static field gradients, which is usually filtered out. Higher frequencies, above 1 kHz in the very low-frequency band, from induction heaters, VDT, and other sources are also filtered out. Some exceptions exist: the portable MultiWave system has flux gate sensors and captures both static magnetic fields and alternating fields up to about 3 kHz; the Positron meter includes a controversial high-frequency 'transient EMF' sensor which detects radio-frequency electric field signals around 5-20 MHz (Deadman et al., 1988); (Heroux, 1991).
In studies in which measurement of personal exposure to magnetic fields is part of the exposure assessment, hip-worn exposure meters are by far the most commonly used. As some investigators have shown that exposure to magnetic fields varies with elevation, it may be desirable to design monitors that could take measurements near potential target organs (when known). Fortunately, monitors on the waist are close to the reproductive organs and the pelvis, the largest reservoir of bone marrow and the presumed origin of leukemia cells.
The efficacy of hip-worn and chest-worn exposure meters for estimating exposure of the whole body and head was examined in detail by DelPizzo (DelPizzo, 1993). The participants wore six exposure meters at a time, positioned at various locations on the body. Over a 2-h period, they engaged in one of the following activities: office work (without a VDT), work on a VDT, mechanical work (excluding welding), and electronics work. While DelPizzo found that hip-worn exposure meters consistently underestimated whole-body average and head exposure, the measurements were highly correlated (R = 0.91 for whole body average, R = 0.83 for head exposure). Chest-worn exposure meters provided better estimates of whole-body and head exposure than those worn on the hip.
In exposure assessment, a way must be found to summarize the characteristics of exposure across time, within groups of individuals, and between many exposure environments. A variety of exposure metrics has been developed to capture the various physical aspects of fields and to provide a summary measure of exposure characteristics that can be aggregated across individual measurements.
A complete description of EMF is possible only with vector mathematics to describe the magnitude and direction in space of the fields over time. A large variety of summary variables can be used to describe a time-varying EMF vector. These variables, called exposure metrics, are intended to capture some particular aspect of the field; however, at this time there is great uncertainty about which exposure metrics (if any) are related to biological responses, and studies have been based mainly on the TWA magnetic field. Some studies also have measured the magnitude of the electric field, and others have explored exposure metrics related to the TWA, such as the geometric mean or percent time exposed to > 0.2-T threshold, but not in great detail. Consequently, the problem of defining and measuring a biologically relevant exposure to EMF is complex and largely unresolved.
Valberg summarized many of the features of exposure to magnetic fields that can be considered in defining exposure metrics (Valberg et al., 1995). He identified some 18 field attributes related to exposure in laboratory experiments (see below), but the list is also applicable to human exposure assessment. The attributes fall into four major categories: (1) characteristics of exposure intensity and timing, (2) frequency domain characteristics, (3) spatial characteristics, and (4) combined EMF exposure characteristics. The first category describes the magnitude and history of exposure, which is most often the focus of studies of exposure to EMF . The second category describes the frequency domain aspects of exposure, such as harmonic content, intermittence, and switching transients, which have been poorly characterized in most cases. The third category describes the characteristics of the vector's spatial orientation, such as field polarization (periodic rotation of the vector) and the spatial uniformity of the fields. The fourth category describes the characteristics arising from combined vector fields, such as the joint occurrence or orientation of alternating and static fields or the joint occurrence of electric and magnetic fields. These four categories represent a hierarchy of increasing complexity in exposure assessment, and increasing levels of mechanistic detail are needed in order to specify an appropriate summary exposure metric.
Despite the uncertainty about biologically meaningful metrics, assessment of exposure to EMF has considerably advanced understanding of the sources and conditions that contribute to EMF in daily life. A variety of factors have been hypothesized to contribute to possible harmful effects of magnetic fields: TWA exposure, percent of time exceeding a certain threshold, frequency harmonics, intermittent exposures, highly variable and constant exposures, and peak exposures are just a few possible metrics considered. To some extent, most time-dependent exposure metrics are correlated with the TWA field magnitude, which is universally measured. Various EMF exposure metrics have been compared in at least two studies, and many of the alternatives were found to be highly correlated with the TWA magnitude of the magnetic field (Armstrong et al., 1990); (Savitz et al., 1994). The high degree of intercorrelation among exposure metrics argues that studies in which TWA field magnitude is used as the primary exposure metric would probably produce similar results, even with another metric of field magnitude; however, these two studies examined only a limited range of exposure metrics that are closely related to the characteristics of exposure magnitude and timing (category 1 above). These exposure metrics can be expected to be well correlated with the TWA field magnitude. No studies have broadly addressed exposure metrics spanning all four of the above categories.
Some examples indicate that exposure metrics for other categories outlined by Valberg may be only weakly correlated and inadequately assessed by relying on the TWA field magnitude. For example, the magnitude of the electric field appears to be largely uncorrelated with the magnitude of the magnetic field in the studies of Armstrong et al. (Armstrong et al., 1990) and Savitz et al. (Savitz et al., 1994), which represent comparisons involving combined EMF exposure characteristics (category 4). Breysse et al. (Breysse et al., 1994b) studied various measures of temporal variability, including first-lag autocorrelation, and found that these exposure metrics ranked the exposures of telephone workers quite differently than the TWA, suggesting a low degree of correlation.
Table 2.2 lists a variety of candidate metrics for exposure to magnetic fields which have been used in epidemiological studies. The Table summarizes the characteristics of each metric in terms of its relation to five attributes of the measured magnetic field: magnitude, frequency content, time variability, spatial characteristics, and relationship to the geomagnetic field. The measures of variation include metrics of both short-term and overall variation, where short-term variation describes some measure of change in field magnitude between two or more successive samples recorded in the data logger. The variation measures are usually dependent on the sampling rate selected for the data logger: higher sampling rates usually show higher overall variation.
A number of epidemiological studies (see Section 4, and Section 2.3.4) have addressed the issue of adverse health effects and exposure to ELF magnetic fields and have used a variety of methods to evaluate power-frequency magnetic fields in residential environments. The primary exposure metric measured has been the average magnitude of the magnetic field. Other surrogate measures of exposure, such as wiring codes and job titles, have also been used. Assessments of past exposure to magnetic fields have focused primarily on the residential environment and on specialized occupations, such as electrical utility workers; however, these jobs are relatively rare in the general population, and far more people are employed in other services, business, or industry, where there may also may be high exposures (NIOSH, 1996).
Four main groupings of exposure environments can be drawn from work on the assessment of exposure to EMF: studies of residential, occupational, school, and transport environments. Together, these environments describe a large cross-section of the US population. Occupational environments have been extensively studied in the context of specific industries and worker populations, particularly the electric utility industry where high exposure to EMF was anticipated. Residential studies have addressed the exposures to children and adults, usually as part of population-based or case-control epidemiological investigations in selected cities. A nationwide survey of residences has been completed that provides a broad overview of sources of EMF in homes. Exposure has also been assessed in schools and transport, but the literature is less extensive.
Most of these studies have a cross-sectional design. In evaluating them, it must be recognized that the results reflect defined study populations over a particular time. Extrapolating the results of one study to other groups or other periods necessarily involves assumptions about the trends over time and how representative the results are for other populations. Various surrogates and measurement techniques may be used, such as job titles, spot readings, personal exposure measurements, and stationary monitoring. These techniques may not always produce comparable estimates of exposure and may have dissimilar errors or bias. Finally, the underlying purpose of the studies may differ: some are designed to characterize the exposures of particular individuals and others to identify and characterize sources, appliances, or unique machinery in an industrial setting. Many studies in the occupational environment are designed to characterize particular jobs or work activities that may be used as surrogates of exposure, such as the exposures of utility lineman or electrical power-line repair maintenance workers.
The concept of homogeneous exposure within groups is the underlying basis for exposure assessment in most epidemiological studies. Ideally, when homogeneous exposure groups exist, all members within the group have an essentially comparable exposure, and all variation in exposure occurs between groups. Although this ideal situation never occurs, it can be approximated. The exposure can be characterized by partitioning the total exposure variation in an analysis of variance (ANOVA) model. The goal of the exposure assessment is to identify a method of grouping individuals in which a large fraction of the total variation is between the groups and a small fraction remains within the groups. Much effort has been devoted over the past 10 years to methods for identifying exposure groupings and applying this concept to exposure surveys (Rappaport et al., 1993).
A frequent problem in epidemiological exposure assessment is defining the characteristics that can be used to define exposure group membership. A variety of historical exposure estimates have been used, such as field readings at some point in time (spot measurements), survey measurements in a residence or workplace, and personal monitoring, but more often other factors, such as work, school, or residential environment, duties performed at a work site, job title, or occupational history, are used as grouping factors. These factors become useful exposure surrogates to the extent that they provide reliable, meaningful partitioning of the exposure variation in the study population.
If measurements have large errors, the surrogates are not specific, or the data are otherwise unreliable, the exposure of study subjects to EMF may be misclassified. Misclassification is often essentially random, leading to switching of individuals between exposure groups. Misclassification may also have a systematic effect that affects certain groups more than others. The effects of random exposure misclassification are usually to decrease the precision of an epidemiological study, making it more difficult to observe an association with disease. When there are multiple exposure groupings, random misclassification can lead to spurious associations in the results (Flegal et al., 1986). The usual effect of systematic misclassification is to bias the risk estimates, possibly creating a specious association or masking a real one.
The main features of the exposure assessment methods used in epidemiological studies can be summarized by three terms: sensitivity, specificity, and potential bias (Lilienfeld & Stolley, 1994). The first two characteristics usually affect individual exposure estimates relative to a group classification, while the last affects group estimates. Sensitivity is the ability of the method to classify correctly an individual who is truly exposed. Specificity refers to the method's ability to classify accurately an individual who is truly not exposed. Bias is the potential for shifting or skewing of exposure estimates away from the true values. Consequently, a biased assessment method may either systematically overestimate or underestimate desired exposure features for groups of individuals in the study population. Bias can be introduced in many ways but most often stems from fundamental limitations of the study design or from limited access to a portion of the study population. Bias can also be introduced by flaws in the sampling strategy used in the exposure assessment, particularly when random sampling or systematic sampling (stratified or cluster sampling) plans are not used.
Considerable emphasis has been placed on the use of personal monitoring for studies of occupational exposure to EMF. Personal monitoring relies on an exposure meter, which is carried on a subject's body to capture spatial and temporal variation in exposure as a series of measurements over time. The individual is the sampling unit, which has the advantage that both within-subject and between-subject exposure variation can be characterized and homogeneous exposure groups can be formed. In some cases, spot measurements may provide a reasonable alternative to personal measurements. In retrospective studies, it is impossible to measure the exposure of the same worker for whom occupational information was gathered; subjects may be untraceable, dead, or inaccessible for reasons of confidentiality or may be in a different exposure circumstance. In such situations, it may be necessary to rely on contemporary measurements of personal exposure from a different, surrogate worker. Contemporary measurements are based on the premise that work practices and sources have remained relatively constant over time. If the spot measurements can capture relevant differences in exposures between jobs, then use of surrogate workers is not necessarily any more meaningful than spot measurements in a work area.
An occupational or work history is a body of data obtained for a study subject, which contains information on the jobs that the subject held throughout his or her working life. Such information is often obtained through questionnaire interviews or databases such as company records. Work histories usually contain information on self-reported job and industry title, company name, a brief job description, how long each job was held, and hours worked per week. In addition, medical records may be obtained from health maintenance organizations, doctors' offices, and disease registries. Death certificates have also been used to obtain information on occupations (Lin et al., 1985).
Job and industry titles are commonly used as surrogates for exposure to EMF and other types of exposure in epidemiological studies. As investigators often have no prior direct measurements of the subjects' past exposures, an entire occupational history must be re-created for each study subject and used to infer past exposure. Usually, researchers cannot obtain individual measurements of exposure for all subjects in a study; consequently, researchers have developed various ways of estimating the exposure of groups of individuals, by re-creating their occupational history and quantifying the related workplace exposures.
Many coding schemes are available, including the Standard Industrial Classification, Standard Occupational Classification (Table 2.3), US census codes,The Dictionary of Occupational Titles, and international occupational codes. Although these codes provide standardized classification methods for jobs or industries, most of these systems were derived for economic or statistical recording purposes and not for the purpose of exposure groupings. Consequently, the coding may not efficiently aggregate similar exposures or even logically classify workers who perform similar tasks and have similar exposures. The Standard Occupational Classification coding system has been widely used in the USA for epidemiological studies. This coding provides a hierarchy of classification that at least tends to keep similar jobs in neighboring groups. This feature is useful when aggregating data from many studies or for clustering jobs held over a working lifetime.
A JEM is a convenient way of organizing data that link job titles or an occupational history to exposure. In a JEM, occupational classification is shown along one axis. Job titles, industry categories, or a combination of these factors are included on this axis. The exposure agent appears across the other axis. Where the rows and columns intersect in the body of the matrix, a level of exposure is displayed. A degree of confidence is often added to the level of exposure, or a percentage of the population exposed may be incorporated. Early versions of JEMs can be traced back to the 1940s, but the first modern JEM was reported in 1980 (Hoar et al., 1980). Although use of JEMs in occupational epidemiology is relatively recent, it has gained wide acceptance in case-control studies (Smith, 1987). The main advantage is the possibility of collapsing many job titles into a smaller number of homogeneous exposure groups. Combining workers into homogeneous groups allows analysis and comparison of data exposure between groups, so that differences in job-exposure and dose-response relationships can be explored. A JEM also can help to economize future research by combining data from many sources; JEMs are often used in hypothesis-generating studies to re-analyze existing data.
Exposure level can be represented in several different ways in a JEM. The most basic JEM simply indicates the presence or absence of a particular exposure for a particular job title. If more information is available, exposure may be ranked, for instance as high, medium, or low. Numerical data from actual measurements is the most desirable source for creating a JEM. In this case, each cell in the JEM ideally contains a group mean value for the exposure metric and some measure of within-group variability.
Although early developments focused on exposure to chemicals, detailed data on personal exposure make it possible to create a JEM for EMF. Today, JEMs incorporating measurements are probably the best approach for assessing exposure to EMF in occupational studies. JEMs allow merging of quantitative measurements with information on job title, industry, and work site to arrive at a grouped estimates of exposure; however, the availability of historically accurate information on exposure to EMF remains a challenge. In general, it is easier to assess past exposures agents such as solvents, because exposure to them is much more memorable to workers or supervisors, and some groups can be identified a priori as having little or no exposure. This is not true for EMF, since virtually everyone is exposed to some degree, and typical exposures are not memorable. In addition, there are usually many more potential sources of EMF than chemicals in a workplace (Savitz, 1993).
An alternative to measuring exposure for a JEM is asking a panel of experts to assign exposure. Such experts should have background knowledge of the tasks in a job, sources of EMF, and specific exposure situations (Loomis et al., 1994a). Job and industry titles may be insufficient to classify the exposures of workers in the absence of specific information on actual work sites. Expert judgment can incorporate this kind of site-specific information, resulting in a better exposure assessment for a particular occupation when information is obtained from a work history or from questionnaires (Post et al., 1991); (Kromhout, 1992). Often, an expert panel is made up of current or former company employees, such as plant workers, supervisors, and industrial hygienists or medical staff.
JEMs used in epidemiological studies of EMF are often specific to a certain industry or location, such as electric power utilities, and may focus solely on occupational exposures. The rational for ignoring residential exposures in occupational studies is the assumption that occupational exposures dominate the total daily or annual exposure to such an extent that other sources are unimportant. This assumption has several potential flaws. First, it presumes that the magnitude of the field is the appropriate measure of disease risk. Second, it assumes that all exposures are additive in time. Third, it assumes that residential sources are much less intense than occupational sources. Fourth, it assumes that the timing of exposure and circadian effects (for example, exposure during sleep, which is not typical on the job) play no role in defining a biologically meaningful exposure. To some extent, all of these assumptions are questionable for EMF, but the last two in particular are not supported by recent studies of EMF. TWA measures of residential exposure can be at least equal to those received in many jobs, and residential appliances can contribute intense (but usually brief) exposure.
Job and industry title can be used as surrogates for exposure to EMF because they allow assumptions to be made about the workers' duties. Certain tasks can be associated with high or low exposure to EMF. For instance, a worker employed as a welder is likely to be exposed to high levels; in contrast, an agricultural worker picking fruit will probably have little exposure to EMF.
Retrospective exposure assessment from job titles can present problems. Workers with the same job title or workers in the same industry today may not be have the same exposures as workers had 20 or 30 years ago, and some job titles that were common in the past may have been rendered obsolete by changing technology. For example, 'keypunch operator' was a common job in the computer industry but has essentially disappeared. Another example is secretarial and administrative work: 30-40 years ago most such workers probably sat at a desk with a telephone and a manual typewriter. Today, it is common for secretaries to have computers, fax machines, and photocopiers within arm's reach of their desks. The addition of these appliances may greatly increase average daily exposure to EMF.
The classifications 'electrical jobs' and 'non-electrical jobs' were developed by Milham to dichotomize workers into exposed and unexposed groups (Milham, 1985). This classification found wide use in early mortality and morbidity studies of EMF. Although this grouping probably leads to considerable misclassification and does not allow derivation of quantitative exposure-response relationships, it makes few assumptions about the nature of exposure to EMF. A three-level classification indicating a degree of exposure to EMF, by background, intermittent, and continuous exposure, was used by Guénel et al. (Guénel et al., 1993).
Measurements of exposure to magnetic field have been collected in a number of occupational studies. Table 2.4 shows the exposures to EMF assigned to different job titles in the occupational epidemiological studies reviewed in this document. The occupations in which exposure to EMF is assumed to be above 'background' levels are listed in order of the arithmetic mean of the TWA magnetic field measured. These exposures have been summarized in a JEM (Yost et al., 1997). When the standard deviations are greater than the arithmetic mean, the distribution is skewed, and the arithmetic mean is not necessarily the best statistic for the central tendency (although many investigators report nothing better). To give perspective, the Table includes magnetic fields measured in some common occupations not considered to involve exposure to EMF and also includes percentiles from a distribution of TWA exposures measured in a population-based study of male workers (Floderus et al., 1996).
Magnetic fields experienced in a variety of categories of jobs, homes, and offices were assessed by Bowman et al. (Bowman et al., 1988) (see Table 2.5). They found significant increase in exposure to EMF in electrical jobs overall. At the work sites examined, spot measurements were taken as close to the worker as possible in the direction of the most likely field sources. In the residences, spot measurements were taken in the center of the living room, bedroom, kitchen, and backyard, under conditions of both low and normal power. The magnetic fields observed at work sites ranged from 0.003 T (microelectronics assembler) to 10 T (electrician in industrial power supply). Only a few measurements were taken in the office environment: one secretary using a VDT had a geometric mean exposure of 0.31 T. Three secretaries who did not use VDTs had a geometric mean exposure of 0.11 T. In the 18 residences measured, a geometric mean exposure of 0.06 T was found.
Using personal exposure meters, Deadman et al. (Deadman et al., 1988) assessed the occupational and residential exposure of a group of electrical utility workers and a comparison background group over one week. The study cohort consisted of 20 workers from six electric utility occupations and 16 workers from two office buildings. Exposures at work and away from work were determined for the two groups. The geometric mean exposure was 1.7 T for the exposed group and 0.16 T for the background group at work and 0.31 T for the exposed and 0.19 T for the background group away from work. The authors proposed that the difference was due to misclassification of some work as non-work. The background group had slightly higher exposure to magnetic fields away from work than at work (0.19 vs. 0.16 T). This difference was presumed to be due to the greater use of hand-held and other electrical appliances at home.
In a study of exposures to magnetic fields in the electric utility work environment (Sahl et al., 1994), exposure to magnetic fields was obtained for 770 work days for the following occupational categories: office staff, meter readers, mechanics, groundmen, lineman, splicers, laborers, plant operators, welders, technicians, machinists, substation operators, and electricians. The fraction of exposures exceeding a 0.5 T summary measure was used to classify occupations into three main groups. The high-exposure group included electricians and substation operators with mean exposures of 2.1 and 1.8 T, respectively; the low-exposure group included office staff, meter readers, and groundmen; and the remaining craft occupations made up the medium-exposure group. The mean exposure for three classifications of office workers (n = 73 workdays) was 0.1, 0.18, and 0.23 T.
Barroetavena et al. (Barroetavena et al., 1994) compared electric and magnetic fields measured at 132 locations in three pulp and paper mills. The median magnetic field strengths in the three facilities were 0.12, 0.33, and 0.15 T, respectively, which were statistically significantly different (p = 0.006), on the basis of the overall electrical consumption at each facility. Differences in measured magnetic fields were also observed in offices at the facilities, with median measurements of 0.05 T in two offices in one facility, 0.18 T in three offices in another facility, and 0.26 T in five offices in the third.
In Denmark, Skotte (Skotte, 1994) studied the 24-h exposure of electric utility workers, office workers, industrial workers, and people living near high power lines to power-frequency electromagnetic fields . A total of 396 measurements were gathered from 301 subjects, 55 of whom were office workers outside the utility companies. The geometric mean exposure observed for office workers was 0.09 T (geometric standard deviation [GSD] 1.8). In normal residences, defined as those not near high-voltage transmission lines, the geometric mean exposure of all study participants was 0.05 T (GSD, 2.1).
Breysse et al. (Breysse et al., 1994b) measured ELF magnetic fields in office environments by taking spot and personal measurements of magnetic field in a large payroll department. Personal exposure was collected for 15 female employees with either an EMDEX C or EMDEX II exposure meter. The spot measurements revealed exposure to 0.13-2.7 T for work with office equipment such as VDTs and photocopiers. The highest measured flux density was attributed to an electrical utility duct. The TWA personal work exposures were 0.1-0.65 T (mean, 0.32 ± 0.15 T).
Burch et al. (Burch et al., 1998) compared exposure to magnetic fields and light with urinary melatonin metabolites in 194 utility workers in northern Colorado, USA. This study is unique because it related exposure to magnetic fields to a short-term change in a human biomarker. Magnetic field and light readings were collected from personal exposure meters for full 24-h periods over five consecutive days. Post-shift and overnight urine samples were used to follow melatonin metabolite excretion. A variety of exposure metrics was used, including a measure of rate of change to describe temporal changes in the field readings and a standardized rate of change metric to measure temporal stability. The standardized metric closely predicted decreased melatonin excretion for both work and night exposures, the values being 0.64 ± 0.04 at work and 0.5 ± 0.04 during sleep for distribution workers and 0.73 ± 0.03 at work and 0.58 ± 0.04 during sleep for office and administrative workers. The TWA exposures over the same intervals showed no association with melatonin, unless combined with the standardized rate of change metric. This result suggests that it may be important to characterize the temporal characteristics of exposure to magnetic fields .
The EMDEX project, conducted by Bracken et al. (Bracken et al., 1995b), was one of the first to document personal exposure for a broad group of utility workers. The exposure of volunteers were collected from utility employees in 13 job classifications at 59 sites in four countries over one year. Uniform sampling procedures and data collection protocols were used at all sites. The volunteers kept diaries of the work and non-work environments they occupied while wearing an exposure meter. Approximately 50 000 h of exposure to magnetic fields taken at 10-s intervals were obtained, about 70% of which were from work environments. Exposure and time spent in environments were analyzed by primary work environment, by occupied environment, and by job classification. The utility-specific job classifications were typically associated with higher exposures; the job classifications with the highest (median workday mean) exposure were substation operators (0.7 T) and electricians (0.5 T). Total variance also tended to be largest for utility-specific job classifications, while the contributions of between-worker and within-worker variation to total variance were about the same. Estimates of time-integrated exposure indicated that in utility-specific job classifications approximately one-half of the total exposure was received on the job. The distributions of non-work exposure were comparable for workers in all job categories, with a median non-workday mean of about 0.09 T.
Savitz et al. (Savitz & Loomis, 1995) studied exposure to magnetic fields in relation to mortality from leukemia and brain cancer among a cohort of 138 905 electric utility workers at five companies in the USA. An extensive personal sampling protocol was used to develop a JEM for exposure reconstruction. Randomly selected workers within occupational categories wore a time-integrating magnetic-field meter (AMEX-3D) so that daily exposure could be estimated for job categories. The mean TWA exposures ranged from 0.12 to 1.27 T for the various categories. Exposure was estimated by linking individual work histories to a JEM based on 2842 work-shift magnetic field measurements. The JEM was optimized to partition variance into between-day (the largest contributor), within occupational categories, and between occupational categories. Kromhout et al. (Kromhout & Loomis, 1997) examined the effectiveness of six alternative grouping strategies for assessing cumulative exposure to magnetic fields in this study population. The quantitative relationship between cumulative exposure to magnetic fields and mortality from brain cancer was sensitive to the choice of grouping scheme, the optimized grouping scheme indicating a stronger relationship than standard grouping based only on job titles.
Thériault et al. (Thériault et al., 1994) conducted a large study of occupational exposure to magnetic fields in relation to cancer risks among three cohorts of electric utility workers in Ontario and Quebec, Canada, and France. A JEM was constructed after monitoring of contemporary occupations to link exposure to 50-60 Hz EMF to the occupational histories of workers at three electric utilities: Electricité de France-Gaz de France, 170 000 men; Ontario Hydro, 31 543 men; and Hydro-Québec, 21, 749 men. Each participant's cumulative exposure to magnetic fields was estimated from measurements of the current personal exposure of 2066 workers who performed tasks similar to those of workers in the cohorts. Past exposure was estimated from knowledge of current loading, work practices, and usage. The median cumulative exposure to magnetic fields was 3.1 T-years, and the 90th percentile was 15.7 T-years.
Baris and Armstrong (Baris et al., 1996b) conducted a substudy to investigate how closely the exposures to magnetic fields based on the last job held compared with exposures based on the workers' entire employment history within the company. The correlations between exposure indices based on the last job and on all jobs varied between 0.75 and 0.78. The mean exposure of all workers was slightly lower when only the last job was considered; however, the last job was particularly useful for identifying the most highly exposed people: the 90th percentile cut-point for the last job had a sensitivity = 0.69, a specificity = 0.97, and a concordance = 0.66 in comparison with all jobs held. These results indicate that use only of the last job to classify exposures results in a greater loss of information than a complete history. Although not all workers starting in highly exposed jobs stayed in them, those who ended their working life in highly exposed jobs had been in these jobs for an extended period. [This finding may be limited to work within the same company.]
Floderus et al. (Floderus et al., 1996) conducted a large population-based study of occupational exposure to EMF (50 Hz) in relation to adult leukemia in Sweden. The exposure assessment was based on a JEM derived from extensive measurements of personal exposure in 1015 workplaces. The JEM derived from these data covered 100 of the commonest occupations in Sweden, with a minimum of four measurements for each occupation. The median workday mean was 0.17 µT, and the 95th percentile was 0.66 µT. Median workday means for occupations with low exposure were 0.04 µT for earth-mover operators and 0.05 µT for concrete workers; the corresponding value for electrical and electronics engineers and technicians and welders was 0.19 µT. Overall, the workday mean in the population was 0.28 µT with a standard deviation of 0.62. This data set is perhaps the most extensive to date for exposure of the general population to EMF.
The validity of various exposure surrogates for describing exposure to EMF in office VDT users was examined by Abdollahzadeh et al. (Abdollahzadeh et al., 1996). They compared 8-h TWA personal exposures to 30-1000 Hz magnetic fields at waist level with a variety of surrogates including: measurements of magnetic fields at 40-1000 Hz at a fixed distance from the VDTs; reported hours of VDT use; and reported distance between the VDT and the subject's waist. The results showed a weak correlation between the 8-h TWA exposure of a VDT user and the magnetic field measured 46 cm from the VDT (R = 0.52, n = 67, p < 0.001). They found no association between self-reported hours of VDT use or self-reported distance between waist and VDT and the average magnetic field. Moreover, individuals' average exposure to magnetic fields did not seem to be affected by other variables such as the position of the VDT on the desk, hours of desk use, and the VDT type (color vs. monochrome).
Nair and Zhang (Nair & Zhang, 1995) also examined a variety of exposure metrics for VDTs by a method based on effects function, to determine the extent to which VDTs can be distinguished from other common sources. They found that that VDT exposure may be of consequence if exposure depends on certain types of time variation of the field. Their work demonstrates that the choice of an exposure-response relationship (i.e. the effects function) can determine if a source will make a small or large contribution to the total exposure.
A study by Lindbohm et al. (Lindbohm et al., 1992) of exposure to VDTs in connection with spontaneous abortions incorporated both measurements and exposure surrogates. They assessed the type of VDT used and the duration of use by questionnaires and employer information. ELF and VLF magnetic field measurements on a representative sample of VDT units in a laboratory provided the exposure information. A relatively high threshold of 0.4 T (ELF) was used for VDT readings to define the low-exposure group; the high-exposure group was defined as receiving > 0.9 T. This grouping scheme made it likely that VDTs would be a strong contributor to exposures, although validation could not be made with personal exposure readings. Electric fields were measured with an active dipole antenna which had a frequency response extending up to 10 Mhz (see Table 2.1). The electric fields 30 cm from the screen were in the range 1.8-22 V/m.
These studies point out the difficulties of conducting a study of VDT operators due to the many possible sources of exposure and the problems in defining an exposure metric that captures the unique characteristics of VDTs.
Wire coding is also a historically stable metric because the type of power distribution lines outside a house do not in general change much over the years. This method is often used in retrospective studies, in which historical stability is important; however, other types of error such as misclassification of wires may be introduced. Wire coding may also introduce confounding or bias that has not been fully understood. Researchers have continued to measure residential exposures with methods such as wire coding, despite the limitations of this method and the modest association with measurements of magnetic fields taken in residences. Wire coding does not provide an estimate of exposure to electric fields within homes (Savitz, 1993).
Table 2.6 shows the distribution of wire codes from seven studies conducted in the USA over the past 15 years. The prevalence of homes with VHCC varies markedly across the studies, from 3% in Denver to 12% in Los Angeles. As some studies excluded underground wiring in homes, the proportions in the remaining categories are somewhat inflated. Table 2.7 shows measured magnetic fields with wire code categories in six studies conducted in the USA since 1982. Measures of central tendency were selected from those available in the studies. Considerable variation can be seen in the measures in each category and particularly in the highest VHCC wire code category, probably reflecting the varying ability of wire codes to capture higher exposures. Table 2.8 shows the percentage of homes in various wire code categories with measured values above a 0.2 or 0.3 µT threshold and indicates that the usefulness of wire codes for identifying high field values in homes varies widely. The proportions in categories in studies from which underground homes were excluded should be interpreted with caution since they are inflated relative to the others.
DelPizzo et al. (Delpizzo et al., 1991) tested the usefulness of spot measurements for classifying residential levels of magnetic fields. Spot measurements were compared with data collected from stationary 24-h monitors. Homes with mean 24-h magnetic fields > 0.075 T were classified as exposed, and those with mean levels < 0.075 T were classified as unexposed. They found that a single spot measurement had at least an 80% chance of classifying a house in agreement with the classification based on the 24-h mean magnetic field and concluded that a small number of readings collected manually over several points within a home can serve to characterize the magnetic field as well as stationary monitoring. DelPizzo and Salzberg (Delpizzo & Salzberg, 1992) found that averaging four or five spot measurement readings over time instead of using a single point in time measurements resulted in a dramatic improvement in the observed-to-true ratio for classifying residential fields; however, these studies were limited because the stationary 24-h measurements in the homes were used as the measure of 'true' exposure. The authors did not measure personal exposures and could not assess the effects of personal activity and use of appliances on exposure classification.
Spot measurements were the basis of a standardized protocol for measuring magnetic fields in homes for a large study of reproductive toxicity conducted in northern California (Yost et al., 1992). A pilot study, which involved taking 252 spot measurements in 24 San Francisco Bay Area homes, was conducted to identify an appropriate sampling strategy. Measurements were taken in multiple locations (center, front right, front left, back right, and back left) in the kitchen, living room, and bedroom of each home, under both low-power (all electrical devices turned off/unplugged) and normal-power conditions. They found that the center normal-power spot measurement was representative of those in other locations. In addition, 79% of the variation in home spot measurements was due to differences between homes (p < 0.00001). The differences between rooms were also significant (p < 0.01). In this protocol spot measurements were taken at the front door and in the center of the kitchen, living room, and bedroom under normal-power conditions. Under that protocol, no more than 45% (and probably considerably less) of the variance would result from within homes. The authors pointed out that London et al. (London et al., 1991), using a similar spot measurement protocol, reported that 19% of the variance was within homes.
In an earlier study, Silva et al. (Silva et al., 1989) reported the spatial distributions of the vertical magnetic field in five types of room found in residences: living rooms, dining rooms, bedrooms, kitchens, and bathrooms. Scatter plots of the vertical field component in the various rooms showed correlation coefficients between center-of-room measurements and elsewhere within the same room that ranged from 0.64 to near 0.8. Although measurements were performed in 81 residences, they were limited because only a single field component was recorded.
The Electric Power Research Institute (EPRI) conducted a survey of 996 residences to determine the levels and sources of residential power-frequency magnetic fields (Zaffanella, 1993). The survey, often called the 'EPRI 1000 homes study', involved a random two-stage cluster sampling plan to achieve a statistically representative sample of EPRI utility customers nationwide. This unique survey, although not designed to describe individual exposures, provides a snapshot of residential fields and the results are probably reasonably representative of residential conditions. An extensive measurement protocol was used, including spot measurements inside the rooms, field recordings in the home, Wertheimer-Leeper wiring codes, measurements of field profiles from wiring outside the home, measurements of household appliances, and measurement of fields from currents in the electrical grounding system. The overall average spot magnitude of the magnetic field inside the surveyed residences was 0.09 T. The median value for the average spot magnetic field reading was 0.06 T and exceeded 0.29 T in 5% of all measured residences. The survey results were corrected by reference to the sample base population representative of national residences. About 28% (95% CI = 22-34) of the homes nationwide exceeded an average interior magnetic field magnitude of 0.1 µT, about 3.3% (95% CI = 1.7-5%) exceeded 0.25 µT, and 0.3% (95% CI = 0.1-0.6) of residences exceeded 0.5 µT.
The 1000 homes study included extensive engineering investigations to identify possible determinants of residential magnetic fields. In most residences, currents in outside power lines and currents flowing in the electrical grounding system were the dominant contributors. Power lines contributed most to the background average magnitude of the magnetic field distributed over the entire residence over the course of a day. Thus, power lines were identified as a significant source of the background fields in the home environment. Currents flowing in the electrical grounding system, in contrast, produced larger variations in magnetic fields over space and time. In some cases, specific features of the electrical system in the residence could be linked to higher magnetic fields: grounding of electrical sub-panels contributed in 4.6% of residences, multiple three-way switches in 5.2% of homes, electric ceiling heat in 2% of homes, and old-style wiring (knob and tube wiring) in 7% of homes. Other more general characteristics of the homes were also associated with higher fields. For example, fields were typically higher in older residences, homes with grounding to a metallic water line, and in duplex or apartment residences. Factors found to be unrelated to interior magnetic fields were household electric energy consumption, construction materials, presence of electric heating (other than radiant ceiling heat), and the presence of children in the home.
One goal of the survey was to evaluate various measurement methods to reliably classify residences with regard to interior magnetic fields. Previous studies had involved a variety of protocols, such as 24-h recordings and spot measurements taken in several rooms, to measure magnetic fields. One comparison of considerable interest concerns the usefulness of spot magnetic field measurements to correctly identify high-field residences. The protocol of the 1000 homes survey was similar to the California protocol described above, with spot measurements taken at the center of several rooms in the home. In the 1000 homes survey, 24-h measurements were also made of both power-line fields and grounding-system fields and they were combined to estimate median fields in the residences. The results are shown in Table 2.9. Remarkably, the median spot readings obtained with the two methods agree quite well. This shows that spot measurements could be used as a first approximation for characterizing magnetic fields in homes.
Table 2.9. Estimated median magnetic fields in the 1000 homes survey
|
|
|
|
||||
|
|
|
|
|
|||
|
|
|
|||||
|
|
0.07 | 0.05 | 0.11 | 0.06 | 0.05 |
|
|
|
0.12 | 0.1 | 0.21 | 0.11 | 0.1 |
|
|
|
0.24 | 0.2 | 0.38 | 0.21 | 0.17 |
|
|
|
0.35 | 0.29 | 0.56 | 0.3 | 0.26 |
|
|
|
0.64 | 0.77 | 1.22 | 0.66 | 0.58 |
|
Kavet et al. (Kavet et al., 1992) assessed the exposure of adults in Maine who lived either near or far from overhead transmission lines. The assessment included 24-h personal exposure measurements, spot measurements in three rooms of every residence, and a 24-h fixed location bedroom measurement. They found greater home and total exposure for subjects residing near highly loaded transmission lines than for subjects living far away from power lines. Both the room spot measurements and 24-h fixed-site bedroom measurement were correlated with home exposure (R = 0.70 and 0.68, respectively). Similarly, in another residential study, Kaune et al. (Kaune et al., 1987) found that spot and 24-h magnetic field measurements were associated, with a correlation coefficient of 0.5.
Kaune et al. (Kaune et al., 1994)also studied 29 children four months to eight years of age to determine whether area (spot and/or 24-h) measurements of power-frequency magnetic fields in residences and schools could be used to predict measured 24-h personal exposures. The average 24-h personal exposure observed was 0.1 T (geometric mean). Greater variation between subjects was found for home exposure than for school exposure. The TWA spot measurements in the home were highly correlated with residential personal exposure (R = 0.9). On the basis of these findings, they established a protocol for measuring residential exposures to magnetic fields. This protocol, like the California protocol developed by Yost et al. (Yost et al., 1992) calls for the following measurements: spot measurements inside the home (center of the subject's bedroom, kitchen, and one other room occupied most frequently by the subject), spot measurements taken immediately outside the front door, and a 24-h fixed measurement in the subject's bedroom.
The repeatability of assessments of residential magnetic fields and wiring codes was examined by Dovan et al. (Dovan et al., 1993) in a study widely known as the 'back to Denver' study. The purpose of the study was to evaluate the long-term stability of wire codes and residential spot magnetic field readings in classifying residential magnetic fields. Wiring code and magnetic field measurements obtained in Colorado homes in 1985 as part of the Savitz study were compared with measurements taken more than five years later. The wire code measurements were in agreement for 73 of 81 homes (90%), and the correlation between spot magnetic field measurements taken in 56 homes in 1985 and measurements taken in 1990 was R = 0.7. A diurnal trend was observed when the average home spot measurements were compared over 24 h, the highest magnetic fields being observed in the late afternoon or early evening and the lowest in the early morning.
London et al. (London et al., 1991) investigated the relationship between childhood leukemia and measurements of EMF in homes or exposure assessed by surrogates such as wiring configurations and self-reported use of appliances in Los Angeles County, California. They recorded detailed measurements of the magnetic field in the child's bedroom over more than 24 h (164 cases and 144 controls), spot measurements of EMF (140 cases and 109 controls), and wiring configurations. The 24-h average magnetic field recorded for the controls was reported as 0.12 ± 0.16 µT and the 90th percentile was 0.19 ± 0.3 µT. The average electric field in the bedrooms of controls was reported as 8 ± 12 V/m. A gradient in the average magnetic field readings was observed for increasing wiring configuration categories: homes with underground wiring, 0.05 µT, and VHCC homes, 0.12 µT. The measurements were similar to those reported by Savitz in Denver, but the prevalence of VHCC homes was higher (11% of control homes in Los Angeles and 3.1% in Denver).
The correlation between magnetic fields and exposure over time was also examined by Kaune and Zaffanella (Kaune & Zaffanella, 1994). Their exposure assessment incorporated spot measurements, stationary 24-h measurements in two locations, and personal exposure measurements for 35 children living in western Massachusetts and northern California. Measurements were taken in the spring of 1990 and again in the winter of 1990-1991. They found a poor correlation between personal exposure measured at the two times (R = 0.1) but fair correlations between spot measurements repeated twice (R = 0.7) and between stationary 24-h measurements repeated twice (R = 0.8).
Kleinerman et al. (Kleinerman et al., 1997) reported on assessment of exposure to magnetic fields for a nine-state residential study of childhood leukemia. Residential magnetic fields were measured in 1354 current and former homes of cases and controls in the study . The TWA magnitude of the magnetic field weighted by the length of time the subject lived in each home was the main exposure metric. The TWA for subjects was estimated by a weighted average of the 24-h bedroom reading with spot readings taken in other rooms. They found that 24-h bedroom measurements adequately characterized the residential exposure of children and that measurements in other rooms contribute only slightly . The mean value for the TWA magnetic field in the homes was 0.11 T, with a standard deviation of 0.11. All of the spot readings were highly correlated with the 24-h bedroom average; the rank correlations ranged from 0.83 in the bedroom to 0.66 for kitchen locations. Front-door spot measurements provided useful information when interior measurements were missing. The rank correlation of the front-door spot reading in comparison with the 24-h reading in the child's bedroom was 0.72; this improved to 0.79 when compared with the estimated TWA for the whole residence. [This study indicates that contemporary spot measurements or front-door readings are reasonably reliable predictors of other contemporary measures of residential magnetic field magnitude.]
Friedman et al. (Friedman et al., 1996) provided the basis for the residential magnetic field survey methods reported by Kleinerman. They compared 24-h stationary measurements in a bedroom with personal exposure measurements for 64 children aged 2-14 years during a typical weekday. The information recorded in activity diaries indicated that the children spent more than 40% of the 24 h in their bedrooms and 68% of their time at home. For children under nine, the levels of exposure at home were highly correlated with total personal exposure (R = 0.94); the correlation was lower in older children (R = 0.59). The 24-h bedroom measures correlated well with personal exposure at home (R = 0.76) for all of the children combined. [These results indicate that 24-h bedroom measurement is a good predictor of both residential and total personal exposure, particularly for younger children.]
Zaffanella et al. (Zaffanella & Kalton, 1998) made a US nationwide random-sample survey of 1000 individuals to provide a more comprehensive picture of exposure to magnetic fields. This study is the first serious effort to evaluate a cross-section of exposure to magnetic fields in the general population. Although somewhat limited by the response rates and potential participation bias, the study provides valuable insight into total exposure to magnetic fields. The preliminary results of this survey were released at a symposium on engineering research into magnetic fields organized by the DOE. The subjects for the survey were recruited by telephone, and those who agreed to participate were mailed a packet with instructions, a time-activity diary, and a personal exposure meter that recorded the magnetic field resultant at a 0.5-s sampling rate. The following conclusions were drawn from the interim analysis of 853 individuals:
The distribution of 24-h TWA exposure in the population is approximately log-normal with a geometric mean of 0.09 µT (95% CI, 0.085-0.096) and a geometric standard deviation of 2.2 (95% CI, 2.1-2.3).
Approximately 15% (95% CI, 12-18%) of the population was estimated to be have 24-h TWA exposure exceeding 0.2 µT, about 2.4% (95% CI, 1.5-3.9%) to have exposures exceeding 0.5 µT, and about 0.4% to have exposures exceeding 1.0 µT. The last value indicates that about 1 million people in the USA have an average 24-h exposure greater than 1.0 µT.
Some variation in 24-h exposures was found by age: the geometric mean exposure for working-age people was about 0.1 µT, and that for retirement-age people was 0.08 µT. The geometric mean exposure for school-age children was about 0.08 µT, and that for pre-school children, 0.06 µT.
About 0.5% of the population have an estimated maximum (peak) exposure to magnetic fields of 100 µT.
The Swedish study of transmission-line fields represented an important advance over previous studies that were based on distribution-line wiring configurations in that it provides a method for estimating historical fields. The calculations are based on established laws of physics and on available physical and operational data rather than on empirical classifications, as for the Denver wiring codes. The calculation method can not be reliably applied to distribution lines because of fundamental differences in the design and operation of the lines and the lack of historical data on load for distribution lines. Consequently, only the magnetic field contribution of the transmission lines can be reliably evaluated. Feychting et al. (Feychting & Ahlbom, 1993) applied the magnetic field calculation method to distribution lines near an unreported number of residences where the distribution line was judged to be a potentially important source of exposure to magnetic fields. [The questionable validity of the calculated field levels near distribution lines may have contributed to some of the inconsistency between contemporary measurements and contemporary calculations discussed above.]
Feychting and Ahlbom (Feychting & Ahlbom, 1994) conducted a study of adult cancers in relation to calculated historical fields. The exposure assessment method was virtually identical to that used by the same authors in their study of childhood cancer (Feychting & Ahlbom, 1993), except that one of the dose metrics calculated was cumulative exposure during the 15 years before diagnosis.
Li et al. (Li et al., 1997) conducted a study of adult cancers in relation to calculated historical fields in Taiwan. They considered in some detail the locations of transmission lines (five voltage categories, from 69 to 345 kV) and homes, with distance readings derived from maps. The stated distance resolution was ± 10 m. The exposure fields were calculated from formulas based on the Biot-Savart law, accounting for line height, phasing, and other factors. No adjustment was made for local distribution lines or local sources, although for apartments the assumed building height was raised to 15 m. The historical average annual load currents, distances from homes to conductors, height of conductors, current phase, and geographic resistivity were provided by the Department of Transmission and Substation Project. Data on the resolution and accuracy of the line current used for historical measurements were not provided. The model calculations were validated by comparing contemporary calculations and measurements, with mixed results: with fields partitioned into three categories < 0.1, 0.1-0.2, and > 0.2 µT, the comparisons showed a concordance of 0.64 between the two exposure estimates. Measurements and calculations for the category > 0.2 µT, with cut-points 0.5 and 1 µT had a concordance of 0.82. The model calculations appeared to have the best predictive value for the highest exposure categories. [The discrepancy between calculations and measurements may be due in part to a contribution of local magnetic field sources to the field, but no indication was provided that the discrepancy is due to the presence of measured fields that were too high or too low. The ± 10-m precision of distance could have had a significant impact on calculations for residences within 20 m of the power lines but would contribute less error for points further away from the transmission line.]
Valjus et al. (Valjus et al., 1995) conducted a detailed analysis of historical field modeling calculations for a study of cancer in Finland. They examined the uncertainty in model calculated fields from transmission lines with considerable thoroughness. For example, the error distributions of power-line and building locations, hourly measurement records of load currents, tower dimensions, variations in conductor height, phase of currents, non-parallel lines, and unbalanced currents were considered in a Monte Carlo analysis. The estimated precision of historical load data was examined by comparison of the estimate to currents calculated from power measurements. The estimate and the calculated value were highly correlated (R2 ~ 0.85). The resolution reported for distance was ± 10 m. [Despite the completeness of the analysis, it is remarkable that no measurements of the magnetic field were performed as part of a verification process for the calculations.]
Olsen et al. (Olsen et al., 1993) calculated magnetic fields to estimate human exposure from power lines and substations for a study of cancer in Denmark. The input parameters for the calculation include distance of the dwelling from source, type of line, dates of construction and reconstruction, average current for year, and ordering phases. [The problems with this study include: only estimates of historical load currents were available, which were provided by experts experienced in the planning and operation of the Danish transmission system; there was no experimental verification of the calculations; and the geometry of substations is much more complicated than that of transmission lines, entailing greater uncertainties. The use of experts is probably not a serious flaw, since they probably had information on historical annual currents from planning surveys.]
In a follow-up analysis of their study of childhood leukemia, Feychting et al. (Feychting et al., 1996) investigated the importance of short-term variability in the time interval of measurement and other factors in residential exposure assessment. They evaluated the validity of contemporary spot measurements and the relative importance of distance from power transmission lines, and, when estimating past exposure to magnetic fields, calculated them with a computer model. Spot measurements were taken 5-31 years after diagnosis, with a median of 16 years. Their study showed that distance was not a simple surrogate for exposure, as first suggested. The relative risks for measurements at the time of the study (contemporary annual average fields, spot calculations, and spot measurements) were all close to or below unity. Neutra and DelPizzo (Neutra & DelPizzo, 1996) noted that spot readings appeared to have poor sensitivity, specificity, and predictive value, even though historical fields were reasonably well correlated with contemporary spot readings (R = 0.7).
[Together, these studies suggest that it is important to account for the historical relationship between exposure and disease outcomes. Contemporary spot or daily readings may introduce enough random and systematic error to obscure or enhance a possible association with disease risk.]
Zaffanella et al. (Zaffanella et al., 1997) studied the use of computer modeling to estimate residential exposures, which could be useful for assessing exposure when access to residences is not possible or when planning a residential development. They used the RESICALC computer program to model magnetic fields due to currents on arbitrary configurations of electric transmission lines, primary and secondary distribution lines, and ground-return currents in neighborhoods based on residential loads and impedance. Experiments conducted at the Magnetic Field Research Facility in Lenox, Massachusetts, simulated a residential electric distribution system. The results showed that the program could accurately model magnetic fields from both supply and ground currents. In some cases, the estimated fields were sensitive to impedance values assigned to the ground network. [Computer modeling for distribution lines requires intensive effort for input data collection, such as careful mapping of power lines, residential coordinates, acquiring load, and grounding data. These input values are critical if the model is to give valid estimates of exposure; however, because these input data are not routinely available and would require special instrumentation to be installed by the electric utility, widespread use of this computer model would be difficult.]
Bowman et al. (Bowman et al., 1997) studied magnetic fields in residences using a physically based multipole model. The model parameters were determined by nonlinear regression techniques in order to fit the 24-h magnitude of the magnetic field recorded in a child's bedroom. The predictions were better correlated with the bedroom readings (R = 0.4) than with Wertheimer-Leeper wire codes (R = 0.27). [Since this model has not been tested in other locations, its generalizability is unknown.]
Kheifets et al. (Kheifets et al., 1997c) also reviewed the historical stability of spot measurements and concluded that they are sufficiently stable over a period of five years to make them suitable for estimating past exposure. In another data set, they examined the usefulness of various surrogates (wire codes, stationary measurements, spot measurements, and personal exposure measurements) for estimating personal exposure measured approximately four months earlier. Contemporary two-day personal exposure measurements were the best indicator of personal exposure, and wire codes were the poorest. The percent variability in exposure explained by the surrogate was 15% for wire codes, 46% for 24-h recordings, 54% for spot readings, and 66% for personal exposures. Contemporary spot and 24-h stationary measurements were similarly effective for estimating past exposure and intermediate between contemporary personal exposure and wire codes in effectiveness. The authors concluded that the potential for exposure misclassification when using wire codes is similar to or greater than that for contemporaneously measured magnetic fields.
Tarone et al. (Tarone et al., 1998) examined the relationship between wire code category and 24-h magnetic field measurements on a state-by-state basis over a nine-state study area. There were insufficient data from Wisconsin for its inclusion in the analysis. More mean measured fields were in the VHCC category than in other categories in six states; two states in which there was no strong trend for increasing fields with wire code (Michigan and Minnesota) were among those with the fewest VHCC homes (four and three homes, respectively). Thus, the aberrant relationship between high wire code and field is probably a result of random variation due to small numbers. [This conclusion is supported by the observation that in the other state with few VHCC homes, the highest mean field was found in those homes.]
Tarone et al. (Tarone et al., 1998) also looked at the distribution of homes with different wire codes, the distribution of mean and median fields within wire codes, and the percentage of homes with exposure > 0.2 and 0.3 µT within a wire code category. While wire codes did not differentiate among measured values, as in the study of Savitz, they were more effective than in the London study. The effectiveness of using wire codes was comparable to or better than that for measured data in other areas examined by Kheifets et al. (Kheifets et al., 1997c). Tarone et al. (Tarone et al., 1998) also looked at the reliability of wire coding in a subgroup of homes where replicate wire coding was done for quality control. Inconsistent determinations were reported for 15 of the 187 homes examined (8%). Of the discrepancies, seven involved distance and only two of the inconsistencies involved VHCC homes.
Electrified mass transit systems are found in many US cities. A US Department of Transportation study (USDT, 1993) of electrified transport systems showed that the average ELF magnetic fields in passenger coaches of trains ranged from approximately 0.5 µT in diesel-powered trains to 13.4 µT in electric-powered trains operating between Washington DC and New York. The maximum fields were found to be approximately five times larger than the average fields. Magnetic fields within the passenger coaches of mass transit systems (subways, trolleys, light rail transit systems) were highly dependent on the vehicle propulsion control system. The average magnetic fields in the passenger coaches of most trolleys and subways were 0.3-0.9 µT, but one system was found to have an average field of 17.8 µT. The principal frequency of the magnetic fields in most transport systems was other than 60 Hz, and, for many systems, the principal field components were at frequencies less than 50 Hz. Consequently, personal exposure measurements with existing exposure monitors do not accurately assess exposure to ELF magnetic fields. The electric fields were small within the coaches of all transport systems tested. Electric fields from external power supply circuits did not significantly penetrate the metallic passenger compartments. The magnitude of EMF in the drivers' compartments of the transport vehicles examined was generally comparable to or lower than the average fields within the passenger coaches.
Sun et al. (Sun et al., 1995) conducted a survey of EMF in 79 schools in Canada for the Carlton Board of Education. They found that the typical magnitude of the magnetic field in classrooms was lower than those in many occupational settings, with a mean of 0.08 µT (Table 2.10). They also attempted to identify possible sources of EMF, such as external wiring and building attributes that contribute to EMF in classrooms. Two-story buildings produced higher fields (geometric mean, 0.08 T ) than did one-story structures (geometric mean, 0.056 µT). Wiring in the floors of classrooms was the most frequently identified local source, while electric typewriters and computers were also common. Outside wiring was a contributing source, but transmission lines were not common enough to be identified as a contributing factor. [Overall, the levels reported in the study were similar to those in many residential and office environments.]
|
|
|
|
|
|
|
|
| Elementary |
|
|
|
|
|
|
| Intermediate |
|
|
|
|
|
|
| Secondary |
|
|
|
|
|
|
| All |
|
|
|
|
|
|
The California Public Health Foundation is performing a statewide measurement survey of EMF in California schools in order to determine the range of EMF in California public schools. Measurements are to be made in a random sampling of about 90 public schools to identify and characterize the sources of the magnetic fields and to evaluate possible mitigation techniques. The measurements will include a survey of classrooms and outdoor activity areas, identification and characterization of magnetic field sources, 24-h field recordings, wire code classification, and identification of nearby outdoor electrical facilities. Preliminary results from the pilot study were presented at the 1996 DOE EMF Contractors Review Meeting in San Antonio, Texas (Neutra et al., 1996). Six schools were involved in the pilot study, and 163 classrooms were measured. Approximately 4% of the classrooms measured in the study were found to have average magnetic field magnitudes > 0.2 T; the median value for these classrooms was about 0.08 T. The commonest overall source of the magnetic fields was ground currents flowing on water pipes or electrical conduits, although for classrooms with fields > 0.2 T outside distribution lines and ground currents contributed about equally to the number of sources observed.
Gauger (Gauger, 1985) studied the magnetic fields from appliances as a function of distance. The levels near hand-held hair-dryers were 0.3-2 µT at 10 cm. Vacuum cleaners, microwave ovens, and small hand-held appliances were identified as projecting the highest fields and/or projecting the furthest distance; 95% of the maximum observed magnetic fields from appliances were < 0.1 T at a distance of 1.5 m.
Mader and Peralta (Mader & Peralta, 1992) demonstrated that the magnitude of magnetic fields drops off at a rate inversely proportional to distance cubed. They presented a method for assessing magnetic fields and a model for predicting the exposure of body extremities. Like Gauger, they found that proximity to the appliance was an important factor. They concluded that appliances do not contribute significantly to whole-body exposure although they may be a dominant source of exposure of the extremities.
Florig and Hoburg (Florig & Hoburg, 1990) modeled and measured magnetic fields from electric blankets. They estimated that the volume-average whole-body exposure for adults was 1.9-2.2 µT; the corresponding values for an eight-year-old child were 2.6-2.7 µT. The magnetic field estimated at the mid-sagittal line 10 cm above the bed was approximately 1 µT. Wilson et al. (Wilson et al., 1996) reported the results of a validation study of a protocol for measuring magnetic fields from electric blankets in homes. The average field over seven spots 10 cm above the bed was 0.45 ± 0.05 µT. The values obtained by Wilson et al. are within a factor of 2 of those reported by Florig and Hoburg. DelPizzo (Delpizzo, 1990) proposed a model for exposure to electric blankets, mattress pads, and other appliances. He suggested that cumulative exposure to magnetic fields of > 400 µT-h per year would be necessary to add significant exposure over background levels.
In the EPRI 1000 homes study, Zaffanella (Zaffanella, 1993) examined a variety of household sources. Appliances were found to produce the highest magnetic fields near the source, but the fields typically decreased rapidly with distance. For example, 13 electric can openers had a median magnetic field magnitude of about 20 T at 20 cm from the source, but this fell to 0.3 T at a distance of 117 cm; microwave ovens had a median power-frequency magnetic field magnitude at 25 cm of 3.7 T, which fell to 1 T at 56 cm. The results for some other appliances in the survey are included in Table 2.11.
|
|
|
|
||||||
|
|
|
|
|
|
|
|||
| Non-ceiling fan |
|
|
|
|
|
|||
| Can opener |
|
|
|
|
|
|
||
| Clock-radio (digital) |
|
|
|
|
|
|
||
| Clock-radio (analog) |
|
|
|
|
|
|
||
| Ceiling fan |
|
|
|
|
|
|
||
| Electric range |
|
|
|
|
|
|
||
| Microwave oven |
|
|
|
|
|
|
||
| Color TV |
|
|
|
|
|
|
||
| Refrigerator |
|
|
|
|
|
|
||
The prototype of a laboratory apparatus for exposure to magnetic fields is a Helmholtz coil, consisting of a pair of circular coils aligned along their open center axis and separated by a distance of one radius. A pair of conductive parallel plates forming an air capacitor is frequently used for exposure to electric fields. Both of these devices produce a reasonably uniform magnetic or electric field around the geometric centerline; the magnitude of the field depends on the physical dimensions, number of turns in the coil, and current (or voltage) applied to the system. In the case of magnetic fields, calibration requires careful attention to construction details and precise control of the current flow in the coils. The uniform region in the center of a Helmholtz coil is rather small, and for large-scale experiments more complex coil designs may have advantages. Kirschvink (Kirschvink, 1992b) described several superior designs with three, four, or five coils which provide highly uniform fields over a large volume. Other exposure systems include solenoids (Merritt et al., 1983; Mullins et al., 1993) or a current sheet to produce uniform magnetic fields.
A common goal in EMF experimentation is to provide a matched control condition that is identical to the exposure in every way except for the desired field exposure. To attain this, a sham exposure is usually set up with an identical apparatus but some modification of the current flow pathway. One method is simply to interrupt the current flow of the Helmholtz pair or the voltage to the parallel plates. This method has the disadvantage that any heat produced by the energized coil is not reproduced in the sham condition. This is not of material concern if the coils are constructed with sufficiently large wire to produce negligible heating. A superior system consists of bifilar windings around the coils, with a parallel pair of insulated wires (Kirschvink, 1992b). In normal operation, parallel currents in the windings yield an external magnetic field. In the sham ('bucked') condition, currents flow in anti-parallel directions, so that the magnetic fields generated by each strand cancel and yield virtually no external magnetic field. The double-wrapped sham produces the same ohmic heating and largely controls for temperature effects. Differences in vibration between the sham and exposed conditions may still occur, but these are usually controlled by careful isolation of the experimental subjects and solid construction of the coils. Identical and interchangeable sham and exposure systems facilitate the conduct of truly double-blind experimental protocols, because the same apparatus can be used for both experimental and control groups, with a simple switch to change the operating conditions.
Another problem encountered in laboratory systems is that stray fields produced by the exposure apparatus or other equipment can contribute to background exposure in the sham controls. Stuchly et al. (Stuchly et al., 1991) devised a system consisting of a quadrupole-coil configuration with four square-wound Merritt coils to minimize stray magnetic fields from the exposure system. The system provides a uniform field within a volume occupied by 16 animal cages and produces a mean flux density of 2 mT which varies by < 10% over the cages. The flux density decreases to < 0.1 µT at 2 m from the coils.
Sometimes, incubators, heaters, motors, and other laboratory equipment can produce large stray fields. To limit these fields, magnetic shielding boxes made of highly permeable materials such as mu-metal may be used. These boxes can reduce stray fields by more than 30-fold and also reduce DC fields. [The biological significance of removing the geomagnetic fields has not been thoroughly studied.] When used inside incubators, shield boxes can degrade temperature control, degrade gas exchange in the culture system, and degrade the field uniformity from theoretical calculated values. Coupling of the magnetic field to the shield box can produce significant mechanical forces, leading to greater vibration in the system.
Some experiments require exposure to combinations of AC and DC fields or control of the magnetic field vector (such as circular polarization) (Shigemitsu et al., 1993). To achieve this, the exposure apparatus is made up of multiple coil windings or sets of orthogonal coils arranged to produce the desired vector components. Careful alignment of the coils and phasing of the currents is needed to produce the desired results (Doynov et al., 1998). [DC magnetic fields are often not controlled in large exposure systems and can vary substantially.]
Another important aspect of laboratory system design is the control of external environmental factors, such as changes in temperature and humidity, light intensity, lighting spectrum, and noise or air-flow distribution inside the animal housing due to air-conditioning equipment. These environmental factors can provide subtle cues to animals or humans and may also alter cell culture conditions. These concerns have led to experimental designs in which assignment between sham and experimental conditions is randomized or counterbalanced. Sometimes it is desirable to randomize cage assignments and periodically rotate cage positions to account for such environmental factors. Light exposure and timing must be controlled especially in experiments involving circadian changes in hormones or behavior. Light intensity, timing, and duration are frequently controlled, but the spectral distribution of the light is often overlooked (Prato et al., 1997).
Careful measurement, spatial mapping, and periodic checking of exposure conditions in the laboratory are necessary for engineering documentation and quality control. Usually, a complete set of engineering measurements is collected before the experiments begin. This includes mapping of field uniformity and measurements of possible frequency harmonics in magnetic field readings. It is also important to characterize any switching transients or other anomalies arising from infrequent operating conditions that may occur during an experiment, such as the effect of opening an incubator door. In the past, there has been strong emphasis on reducing switching events that produce a high rate of change in the magnetic field.
In in vitro experiments with EMF, cultures are routinely exposed to a uniform external magnetic flux density. Initially, many researchers did not measure or estimate the resulting induced electric field strength or current density in the sample medium. The magnitude and spatial distribution of the induced electric field are highly dependent on the sample geometry and the relative orientation of the culture medium with respect to the magnetic field (Misakian, 1997; Misakian & Kaune, 1990). Bassen et al. (Bassen et al., 1992) studied the electric fields induced in several of the most frequently used laboratory culture dishes and flasks under various exposure conditions. They developed a set of simple, quantitative tables to predict the induced electric fields and currents which were based on measurements and calculations of the electric field distributions in the aqueous sample volume subjected to a uniform, sinusoidal magnetic field of known strength and frequency. The electric field and current density can also be calculated numerically from relatively simple but flexible spreadsheet models (Hart, 1996).
These studies highlight the need for careful engineering design and evaluation of laboratory exposure systems, since all laboratory systems have potential strong points and weaknesses and involve engineering compromises. Researchers should understand these design elements in order to use the exposure apparatus to the best advantage. Close collaboration between engineers and laboratory scientists is necessary, and can result in clever adaptations of exposure systems to focus on a desired experimental test. Several funding agencies have made external site reviews for quality control.
Personal exposure has been estimated in the residential setting in order to study children's exposure. Kleinerman (Kleinerman et al., 1997) estimated the exposure of 1633 children < 14 years of age and found that their daily mean exposure was about 0.11 ± 0.11 µT. These values are not based on direct personal monitoring but do attempt to account for total exposure.
Studies of occupational exposure have focused on electrical and utility workers; only recently have data become available on the exposure of the general population. Studies in the general population indicate that the median of the daily mean occupational exposure for adults is about 0.17 µT. Zaffanella et al. (Zaffanella & Kalton, 1998) estimated that the distribution of 24-h TWA exposure in the general US population was log-normal, with a geometric mean of 0.09 µT and a geometric standard deviation of 2.2. Thus, about 15% of the population have 24-h exposures exceeding 0.2 µT, about 2.4% are exposed to > 0.5 µT, and 0.5% to > 1 µT.
When they are practical, direct personal measurements of magnetic fields are generally the preferable method of exposure assessment. Direct measurements provide a quantitative estimate of exposure to a clearly defined field. Even direct measurements, however, may not allay substantial uncertainty about classification of the exposure, as factors such as seasonal variation, changes in work tasks, intermittent use of appliances or tools, changing current loads, and variable proximity to wiring can contribute to large day-to-day variation in measurements. The time of data collection during a day or a season can lead to systematic bias in estimates of daily or annual average exposure. Personal exposure monitors can also be intrusive, so that people may alter their usual activities because they are wearing the meter. Because of the wide variation in exposure to magnetic fields, very many measurements must be made in order to obtain reasonably precise estimates of exposure. It should also be noted that the TWA fails to reflect a large number of potentially relevant exposure parameters, such as time above thresholds, intermittency, and transients.
Many studies of EMF have been based on measurements at one point in time (spot measurements), stationary monitoring over time, or area measurements involving mapping of the spatial characteristics of fields. While offering a quantitative estimate of fields, such measures also lead to substantial uncertainty about exposure classification. These types of measurements have several disadvantages, including the fact that they ignore personal activity patterns such as mobility and use of tools or appliances; they do not reflect past exposure; and they exclude possible parameters of exposure such as specific frequency content, polarization, and static magnetic fields. These types of measure do, however, have the advantage of simplicity and can provide reasonable estimates of human exposure when mobility is restricted to a particular room or residence. With additional equipment, stationary monitoring can be used to capture a wider range of EMF characteristics, providing a greater variety of potential exposure metrics. Contemporary spot measurements are useful for checking the validity and appropriateness of calculations for magnetic fields from power lines in some situations.
The value of contemporary spot measurements as surrogates for past exposure remains uncertain. The limited data indicate that spot measurements are reasonably well correlated (R Å 0.7) with similar measurements over several years. Dovan (Dovan et al., 1993) found that contemporary spot measurements taken within wire code categories remained correlated with home average readings collected five years earlier (R Å 0.7 for low power). Dovan purposely oversampled high-field very high frequency and case homes from the data set of Savitz et al. (Savitz et al., 1988), so this may overstate the predictive value of spot measurements somewhat. The relationship between spot measurements and personal exposure is less clear. Kaune and Zaffanella (Kaune & Zaffanella, 1994) found essentially no correlation over time for the personal exposure of children in residences; Koontz (Koontz et al., 1992), in a study of children's exposure, found a significant correlation over a few days but not across seasons. In residences, the combination of 24-h bedroom measurements with spot measurements in several other rooms appears to be a good method for determining the contemporary TWA household exposures of children. The correlation improves as the age of the child decreases.
Assessment of exposure to EMF for studies of human health effects is difficult because direct measurements often cannot be obtained, particularly for studies of chronic diseases, as the exposure of interest may have occurred years previously, and the actual circumstances of exposure cannot be recreated. In such studies, therefore, all assessments of exposure, including direct measurements, are surrogates for the exposure of interest. The surrogates most widely used are contemporary measurements, job titles, proximity to electrical equipment, calculated historical fields, and wiring configuration coding (wire codes).
Occupational histories are often incomplete and lack sufficient detail on actual work activities for past exposures to be reconstructed. As exposures to EMF are not memorable, questionnaires are of limited value. Contemporary measurements of similar workplaces may account for all sources but may be poor surrogates for past exposures. Classification of exposure on the basis of 'electrical jobs' provides a crude but useful tool for studies of EMF. The wide variation in EMF intensity results in considerable overlap and misclassification. This classification scheme, however, includes few assumptions about the exposure metric used.
An alternative method is use of a job-exposure matrix (JEM) to obtain quantitative estimates of exposure to electric or magnetic fields. In modern occupational studies, the JEM appears to provide the most flexible, stable tool for reconstructing exposure. A JEM can be constructed for almost any desired exposure if measurements are available, although it still relies fundamentally on occupational titles to classify exposure. The absence of complete data on exposures in a wide variety of occupations remains a limitation in studies of occupational exposure.
Many different surrogates for exposure have been used in studies of residential exposure, including wire coding, spot measurements, 24-h bedroom measurements, personal monitoring, and calculations based on physical models. All of these techniques have some limitations, and all of them result in misclassification of exposure. Measurements have the advantage that they capture all sources of exposure. Yet, as noted above, contemporary measurements may be poor predictors of past exposures. Estimates based on wiring configurations or model calculations are of historical value, but these techniques account only for external sources of EMF such as transmission and distribution power lines. These methods also result in misclassification of exposure, perhaps non-randomly, and tend to lead to underestimates of total exposure as many local sources are not taken into account.
The system of wire codes was developed by Wertheimer and Leeper (Wertheimer & Leeper, 1979) to predict residential magnetic fields from the distance and configuration of transmission and distribution lines near residences. The validity of wire codes has been questioned because the different wire code categories for contemporary measured fields overlap widely. Several studies have shown that wire codes can be used consistently to rank homes crudely according to the median magnetic field intensity. Dovan (Dovan et al., 1993) showed that wire codes change little over time, but their usefulness for predicting past exposures remains an open question. The strengths of the wire code method include the following:
In an alternative method for assessing residential exposure, physics-based calculations are used to estimate past fields. Generally, retrospective residential exposure assessment based on calculations of magnetic fields from nearby transmission lines on the basis of historical load currents should be more accurate than either wire codes or contemporaneous measurements, especially for single-family homes sufficiently close to a transmission line to ensure that the fields originated mainly from that source. In those homes, failure to account for fields from local sources should have less impact because transmission line fields dominate over most local field sources. The calculations for distribution lines are less reliable owing to the presence of ground currents and fluctuating loads; however, this method may be better than wire codes. Calculations for apartments, where local field sources might still dominate, are also uncertain. In some cases, the methods of calculation have been validated against contemporary spot measurements, and this has helped to establish the predictive value of the models in study populations.
Calculations of historical magnetic fields are most applicable when the geometry of the power-line sources is relatively simple, e.g. transmission lines, provided there are adequate data on load currents. Limited resolution in the measurement of distance to the residence can dominate the uncertainty in field estimates near the line. Close proximity to high-voltage transmission lines may also be an indication of substantial exposure to both magnetic and electric fields. The availability of high-quality data on load currents is also critical for this approach to succeed, although in some cases it may be possible to obtain reasonable estimates from informed experts. With the deregulation of utilities in the USA, it may become more difficult to obtain data on load currents because of proprietary interests. The strengths associated with modeling historical fields are the possibilities of estimating:
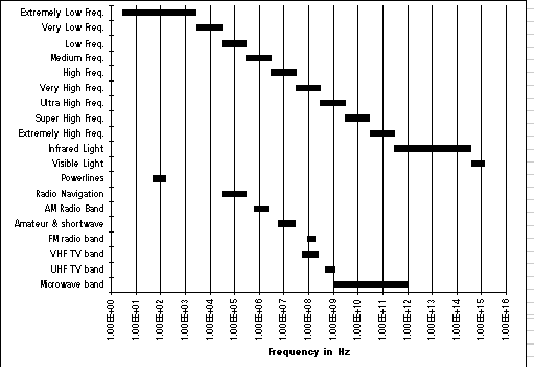
(A) 
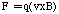 (a)
(a)
(B) 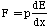
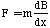 (b)
(b)
(C) 
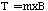 (c)
(c)
(D) 
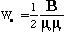 (d)
(d)
(E) 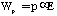
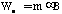 (e)
(e)
For explanation of the "cross-product" in equations (a),
(C) and (c), see Figure 2.2. The "dot-product" or scalar product of two
vectors a and b (equations. (E) and (e)) is equal to ab cos where is the
angle between the vectors a and b.
Figure 2.3 Illustration of "cross-product" (equations (a), (C) and
(c) in Figure 2.2: The resulting vector c = ab is in a direction perpendicular
to the plane defined by a and b and its magnitude is equal to the product
of their mutually perpendicular components: c = ab sin ).
|
|
|
|
|
|
|
|
|
|
|
| Amex |
|
|
|
|
|
|
|
|
|
| Amex-3D |
|
|
|
|
|
|
|
|
|
| Emdex C |
|
|
|
|
|
|
|
|
|
| Emdex II |
|
|
|
|
|
|
|
|
|
| Positron |
|
|
|
|
|
|
|
|
|
| Monitor Ind. |
|
|
|
|
|
|
|
|
|
| Multiwave |
|
|
|
|
|
|
|
|
|
| Power frequency Meter MOD120 |
|
|
|
|
|
|
|
|
|
| STAR |
|
|
|
|
|
|
|
|
|
| MAG 01 |
|
|
|
|
|
|
|
|
|
| IREQ |
|
|
|
|
|
|
|
|
|
| Heitanen & Jokela, 1990 |
|
|
|
|
|
|
|
|
|
| Juutilainen & Saali, 1986b |
|
|
|
|
|
|
|
|
|
| Sydkraft |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| Arithmetic mean (TWA) | TWA | All | Magnitude |
| Geometric mean | GM | All | Magnitude |
| Median (50th percentile) | B50 Med | All | Magnitude |
| Peak (maximum) value | Bpeak | All | Magnitude |
| 99th or Nth upper percentile | B99 | Armstrong | Peak magnitude |
| Percent of time > threshold | Tn | Armstrong | % magnitude > limit |
| Percent of time < threshold | Tn | Armstrong | % magnitude < limit |
| Percent of time in window | Tw | Armstrong | % magnitude > limit1 & < limit2 |
| Total harmonic distortion | THD | Zaffanella 1993, Breysse 1997 | Frequency B-field |
| High frequency electric transients | HFET | Deadman | Frequency, E-Field |
| Average absolute sequential difference | AASD | Breysse 1994 Zaffanella 1993 | Short term variability |
| First lag autocorrelation | Breysse 1994 Thomas 1996 | Short term correlation | |
| Standardized rate of change | RCM | Birch et.al. | Short term correlation |
| Jaggedness index | Jag | Wenzel | Short term variability |
| RMS Rate of change | RC | Wilson et.al. | Short term variability |
| Standard deviation | SD | Armstrong, etc | Time variability |
| Geometric standard deviation | GSD | Armstrong, etc | Time variability |
|
|
|
| 1633 | Electrical and electronic engineers |
| Electrical and electronic technicians | |
| Broadcast equipment operators | |
| Electronic repair, communications & industrial equipment workers | |
| Data processing equipment repairers | |
| Household appliance and power tool repairers | |
| Telephone line installers and repairers | |
| Telephone installers and repairers | |
| Miscellaneous electrical and electronic equipment repairers | |
| Supervisors, electricians and power installers, and repairers | |
| Electricians | |
| Electricians apprentices | |
| Electric power installers and repairers | |
| Power plant operators | |
| Motion picture projectionists | |
| Electric power wire and cable workers | |
| Power station operators | |
| 7714 | Welders and cutters |
| Television and radio repairman | |
| 1636 | Computer engineers |
| 1712 | Computer systems analysts |
| 3650 | Radiological technologists and technicians |
| 3971 | Programmers, business |
| 4732 | Telephone Operators |
| 4752 | Production and planning clerks |
| 6881 | Precision inspectors, testers, and graders |
| 7830 | Production testers |
|
|
|
|
|
|
|
|
|
|
||||
| Engine driver | Railroad | (Floderus et al., 1994)(Sobel et al., 1995)(Sobel et al., 1996) |
|
|
|
| Driver of electric vehicle | Railroad | (Guénel et al., 1993) | Continuous |
|
|
| Railroad engineer | (Lin et al., 1985) | A (definite) |
|
|
|
| Railroad engine driver | (Tynes et al., 1992) | Intermediate |
|
|
|
| Cable joiner and lineman | (Fear et al., 1996) |
|
|
||
| Electric power line installers and repairers | (Milham, 1985)(Deapen & Henderson, 1986)(Demers et al., 1991)(Loomis et al., 1994b)(Savitz et al., 1994)(Spitz & Johnson, 1985)(Nasca et al., 1988)(Wilkins & Wellage, 1996)(Savitz et al., 1998b) |
|
|
||
| Lineman | Electric company | (Lin et al., 1985) | A (definite) |
|
|
| Power-line worker | (Tynes et al., 1992) | Heavy EMF |
|
|
|
| Electrician | So. Cal. Edison | (Sahl et al., 1993) |
|
|
|
| Sewing-machine operators | Garment industry | None |
|
|
|
| Dressmakers, seamstresses and tailors | (Sobel et al., 1995)(Sobel et al., 1996) |
|
|
||
| Dressmakers and tailors | (Coogan et al., 1996) | Low |
|
|
|
| Machinist | So. Cal. Edison | (Sahl et al., 1993) |
|
|
|
| Forestry and logging jobs | NONE |
|
|
||
| Welder | (Lin et al., 1985) | B (probable) |
|
|
|
| Welders and flame cutters | (Milham, 1985)(Deapen & Henderson, 1986)(Demers et al., 1991)(Spitz & Johnson, 1985)(Nasca et al., 1988)(Wilkins & Wellage, 1996) (Rosenbaum et al., 1994) |
|
|
||
| Welders and flame cutters | (Coogan et al., 1996) | Medium |
|
|
|
| Welder | (Sobel et al., 1996)(Sobel et al., 1995) (Davanipour et al., 1997) | High |
|
|
|
| Electrical fitter | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
||
| Electrical and electronic production fitter | (Fear et al., 1996) |
|
|
||
| Electrician | Electric power | (Guénel et al., 1993) | Continuous |
|
|
| Electrician | Industry | (Lin et al., 1985) | A (definite) |
|
|
| Electrician | Steel mill | (Davanipour et al., 1997) | High |
|
|
| Electrician | Manufacturer of electrical machinery | (Guénel et al., 1993) | Continuous |
|
|
| Electrician, power supply | (Tynes et al., 1992) | Heavy EMF |
|
|
|
| Cable splicer | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Power station operator | (Milham, 1985)(Deapen & Henderson, 1986)(Tynes et al., 1992)(Loomis et al., 1994b)(Savitz et al., 1994)(Wilkins & Wellage, 1996)(Fear et al., 1996)(Savitz et al., 1998a) |
|
|
||
| Power plant operator | (Demers et al., 1991) | Group 1 |
|
|
|
| Worker | Sewing factory | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
|
| Relay technician | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Sheet metal worker | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
||
| Technician | Southern California Edison | (Sahl et al., 1993) |
|
|
|
| Electrician | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Power plant operator | Southern California Edison | (Sahl et al., 1993) |
|
|
|
| Lineman | Southern California Edison | (Sahl et al., 1993) |
|
|
|
| Welder | Southern California Edison | (Sahl et al., 1993) |
|
|
|
| Motion picture projectionist | (Milham, 1985)(Deapen & Henderson, 1986)(Loomis et al., 1994b)(Savitz et al., 1994)(Sobel et al., 1996)(Sobel et al., 1995)(Wilkins & Wellage, 1996)(Savitz et al., 1998a) |
|
|
||
| Substation operator | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Welder | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Electric generation plant operator | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Mechanic | Southern California Edison | (Sahl et al., 1993) |
|
|
|
| Machinist | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| 95th percentile of males |
|
||||
| Lineman | 5 US electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Dental hygienist | (Davanipour et al., 1997) | Medium |
|
|
|
| Conductor | Railroad | (Floderus et al., 1994) |
|
|
|
| Railroad assistants and lineman | Railroad | (Floderus et al., 1994) |
|
|
|
| Railroad track walker | (Tynes et al., 1992) |
|
|
|
|
| Tram driver | (Tynes et al., 1992) |
|
|
|
|
| Conductors and motormen, urban rail transit | (Milham, 1985)(Deapen & Henderson, 1986); |
|
|
||
| Electrical and electronics assembler | (Coogan et al., 1996)(Wilkins & Wellage, 1996)(Johnson & Spitz, 1989)(Fear et al., 1996) (Sobel et al., 1996)(Sobel et al., 1995) (Rosenbaum et al., 1994) |
|
|
||
| Employee | Utilities | (Spitz & Johnson, 1985) |
|
|
|
| Employee | Utilities | (Nasca et al., 1988) |
|
|
|
| Electrical and electronic equipment repair | (Loomis et al., 1994b)(Savitz et al., 1994)(Savitz et al., 1998b) |
|
|
||
| Electrical and electronics apparatus mechanics and installers | (Johnson & Spitz, 1989) |
|
|
||
| Electrical equipment repairer | (Coogan et al., 1996) |
|
|
|
|
| Electrical equipment repairman | (Spitz & Johnson, 1985)(Nasca et al., 1988) |
|
|
|
|
| Household appliance and power tool repairers | (Wilkins & Wellage, 1996) |
|
|
||
| Household appliance installers and mechanics | (Deapen & Henderson, 1986) |
|
|
||
| Painter | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Office machine repairer | (Deapen & Henderson, 1986) |
|
|
||
| Lineman | Telephone company | (Lin et al., 1985) |
|
|
|
| Telephone technician | (Demers et al., 1991) |
|
|
|
|
| Mail and message distributing occupations | None |
|
|
||
| Groundskeepers and gardeners | None |
|
0.90 | ||
| Boilermaker | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
1.05 | |
| Service worker | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
0.69 | |
| Serviceman | Electric company | (Lin et al., 1985) | A (definite) |
|
0.69 |
| Instrument and control technicians | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
1.12 | |
| Rigger | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
0.37 | |
| Electriciana | (Sobel et al., 1995) (Sobel et al., 1996) (Davanipour et al., 1997) | Medium |
|
0.32 | |
| Electrician | (Demers et al., 1991) | Group 1 |
|
0.32 | |
| Electrician | Electrician; installation | (Guénel et al., 1993) | Continuous |
|
0.32 |
| Electrician | Non-industrial | (Lin et al., 1985) | B (probable) |
|
0.32 |
| Electrician and apprentice | (Milham, 1985) (Deapen & Henderson, 1986)(Loomis et al., 1994b) |
|
0.32 | ||
| (Savitz et al., 1994)(Spitz & Johnson, 1985) (Johnson & Spitz, 1989)(Nasca et al., 1988)(Wilkins & Wellage, 1996)(Fear et al., 1996)(Rosenbaum et al., 1994)(Savitz et al., 1998b) | |||||
| Electrician, installation | (Tynes et al., 1992) | ||||
| Electrician | (Coogan et al., 1996) | Medium | 0.37 | 0.32 | |
| Factory hand and other unskilled worker | Iron and steel works | (Guénel et al., 1993) | Continuous | 0.36 | 0.43 |
| Factory hand and other unskilled worker | Iron foundries | (Guénel et al., 1993) | Continuous | 0.36 | 0.43 |
| TV and radio repairman | (Milham, 1985)(Deapen & Henderson, 1986)(Tynes et al., 1992)(Fear et al., 1996) | 0.36 | 0.23 | ||
| TV repairer | (Davanipour et al., 1997) | Medium | 0.36 | 0.23 | |
| Repairman for radio, TV, and electronic appliances | (Lin et al., 1985) | B (probable) |
|
|
|
| Household appliance and power tool repairer | (Dennis et al., 1991)(Savitz et al., 1994) |
|
|
||
| Commercial and industrial electronic equipment repairer | (Demers et al., 1991)(Wilkins & Wellage, 1996) | Group 2 |
|
|
|
| Technical worker | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Traffic, shipping, and receiving clerks | NONE |
|
|
||
| Electrical engineering technician | (Milham, 1985)(Deapen & Henderson, 1986)(Loomis et al., 1994b)(Savitz et al., 1994)(Wilkins & Wellage, 1996)(Sobel et al., 1995)(Sobel et al., 1996)(Savitz et al., 1998b) |
|
|
||
| Electrical engineering technician | (Coogan et al., 1996) | High |
|
|
|
| Electrical engineering technician | (Demers et al., 1991) | Group 4 |
|
|
|
| Telecommunication technician | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Electrical and electronic engineers | (Milham, 1985)(Deapen & Henderson, 1986)(Loomis et al., 1994b)(Savitz et al., 1994)(Johnson & Spitz, 1989)(Wilkins & Wellage, 1996)(Savitz et al., 1998b) |
|
|
||
| Electrical and electronic engineers | (Coogan et al., 1996) | High |
|
|
|
| Electrical and electronics engineers | (Demers et al., 1991) | Group 4 |
|
|
|
| Electrical and electronics engineers | Industrial | (Lin et al., 1985) | A (definite) |
|
|
| Electrical and electronic engineers (professional) | (Fear et al., 1996) |
|
|
||
| Electrical engineer (so described) | (Fear et al., 1996) |
|
|
||
| Other electronic maintenance engineers | (Fear et al., 1996) |
|
|
||
| Engineer | Electric power | (Guénel et al., 1993) | Continuous |
|
|
| Engineer | Electric company | (Lin et al., 1985) | A (definite) |
|
|
| Engineer | Telephone company | (Lin et al., 1985) | A (definite) |
|
|
| Telecommunications engineer | (Lin et al., 1985) | A (definite) |
|
|
|
| Maintenance mana | (Lin et al., 1985) | C (possible) |
|
|
|
| AC, heating, and refrigeration repairman | (Deapen & Henderson, 1986) |
|
|
||
| Mechanic, Power plant | (Johnson & Spitz, 1989) |
|
|
||
| Mechanic, utility services | (Johnson & Spitz, 1989) |
|
|
||
| Programmer, systems planner | EDP services | (Guénel et al., 1993) | Continuous |
|
|
| Station master and train dispatcher | Railroad | (Floderus et al., 1994) |
|
|
|
| Electronics wireman | (Fear et al., 1996) |
|
|
||
| Precision inspectors, testers and related workers | (Coogan et al., 1996) | Medium |
|
|
|
| Tool and die maker | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
||
| Pipe coverer | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Farmer | NONE |
|
|
||
| 75th percentile of males |
|
||||
| Electrical equipment salesman | (Spitz & Johnson, 1985) | Broad definition |
|
|
|
| Electrical goods and appliance salesman | (Johnson & Spitz, 1989) |
|
|
||
| Sales occupations, retail | NONE |
|
|
||
| Computer programmer | (Coogan et al., 1996) | Medium |
|
|
|
| Computer programmer | (Loomis et al., 1994b) (Savitz et al., 1998b) |
|
|
||
| Electrician, other | (Tynes et al., 1992) | Intermediate |
|
|
|
| Other electrical worker | (Tynes et al., 1992) | Weak |
|
|
|
| Engineer (nonspecified) | Electric, electronic, aerospace, and tele-communication | (Lin et al., 1985) | B (probable) |
|
|
| Other engineers | (Coogan et al., 1996) | Medium |
|
|
|
| Repairman | Telecommunications | (Lin et al., 1985) | B (probable) |
|
|
| Craft supervisor | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Foreman | Electric company | (Lin et al., 1985) | A (definite) |
|
|
| Supervisors, electricians, and power installers and repairers | (Loomis et al., 1994b)(Savitz et al., 1994) |
|
|
||
| (Wilkins & Wellage, 1996) (Savitz et al., 1998b) | |||||
| Mechanic | 5 U.S. electric utilities | (Savitz & Loomis, 1995) |
|
|
|
| Industrial engineer | (Davanipour et al., 1997) | Medium |
|
|
|
| Food and beverage preparation and service | NONE |
|
|
||
| Carpenter | (Lin et al., 1985) | C (possible) |
|
|
|
| Carpenter | (Davanipour et al., 1997)(Sobel et al., 1996)(Sobel et al., 1995) | Medium |
|
|
|
| Receptionist | NONE |
|
|
||
| Clothing cutter | Garment industry | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
|
| Heavy equipment operator | (Davanipour et al., 1997) | Medium |
|
|
|
| Operating engineer | (Coogan et al., 1996) | Low |
|
|
|
| Woodworking, textile, and shoe machine operators | (Coogan et al., 1996) | Low |
|
|
|
| Computer system engineer | (Davanipour et al., 1997) | Medium |
|
|
|
| Computer systems analysts / scientists | (Coogan et al., 1996) | High |
|
|
|
| Engineering technician | (Coogan et al., 1996) | Medium |
|
|
|
| Telephone fitter | (Fear et al., 1996) |
|
|
||
| Telephone line installers and repairers | (Milham, 1985)(Deapen & Henderson, 1986)(Loomis et al., 1994b)(Savitz et al., 1994)(Wilkins & Wellage, 1996)(Sobel et al., 1996)(Sobel et al., 1995)(Savitz et al., 1998b) |
|
|
||
| Telephone line installers and repairers | (Demers et al., 1991) | Group 1 |
|
|
|
| Supervisors, sales occupations, insurance, real estate, and business services | NONE |
|
|
||
| Computer equipment operator | (Loomis et al., 1994b)(Savitz et al., 1998b) |
|
|
||
| Machine molder | Iron foundries | (Guénel et al., 1993) | Continuous |
|
|
| Printing machine operator | (Coogan et al., 1996) | Low |
|
|
|
| Serviceman | Telephone company | (Lin et al., 1985) | A (definite) |
|
|
| Lathe worker | (Davanipour et al., 1997) | Medium |
|
|
|
| Machinist | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
||
| Machinist | (Lin et al., 1985) | C (possible) |
|
|
|
| Machinists / tool and die makers | (Coogan et al., 1996) | Medium |
|
|
|
| Toolmaker | (Lin et al., 1985) |
|
|
||
| Janitors and cleaners | None |
|
|
||
| 50th percentile of males |
|
||||
| Telephone installer | (Tynes et al., 1992) | Weak |
|
|
|
| Telephone installers and repairers | (Deapen & Henderson, 1986)(Wilkins & Wellage, 1996)(Savitz et al., 1994)(Rosenbaum et al., 1994) |
|
|
||
| Authors and technical writers | (Coogan et al., 1996) | Low |
|
|
|
| Data processing equipment repairer | (Wilkins & Wellage, 1996) |
|
|
||
| EDP equipment repairer | (Coogan et al., 1996) | High |
|
|
|
| Assembler | (Sobel et al., 1996)(Sobel et al., 1995) |
|
|
||
| Assembler | Household appliances | (Deapen & Henderson, 1986) |
|
|
|
| Assembler | Radio, TV and communications equipment | (Deapen & Henderson, 1986) |
|
|
|
| Assembler | Electrical machinery and supplies (NEC) | (Deapen & Henderson, 1986) |
|
|
|
| Assembler | Electrical equipment (not specified) | (Deapen & Henderson, 1986) |
|
|
|
| Chemist | (Davanipour et al., 1997) | Medium |
|
|
|
| Coil winder | (Fear et al., 1996) |
|
|
||
| Data processing machine repairman | (Deapen & Henderson, 1986)(Savitz et al., 1994) |
|
|
||
| Highway patrolman | (Lin et al., 1985) | B (probable) |
|
|
|
| Mechanics, computers and business machines | (Johnson & Spitz, 1989) |
|
|
||
| General office occupations | NONE |
|
|
||
| Teacher | NONE |
|
|
||
| Accountant | (Davanipour et al., 1997) | Medium |
|
|
|
| Accountant | (Coogan et al., 1996) | Low |
|
|
|
| Billing, posting and calculating machine operators | (Coogan et al., 1996) | Medium |
|
|
|
| Dispatcher | (Lin et al., 1985) | B (probable) |
|
|
|
| Dispatcher | (Demers et al., 1991) | Group 3 |
|
|
|
| Air traffic controller | (Demers et al., 1991)(Loomis et al., 1994b)(Savitz et al., 1998b) | Group 3 |
|
|
|
| Broadcast equipment operator | (Demers et al., 1991)(Loomis et al., 1994b)(Savitz et al., 1994)(Wilkins & Wellage, 1996)(Savitz et al., 1998b) | Group 3 |
|
|
|
| Radio/telegraph operator | (Tynes et al., 1992)(Rosenbaum et al., 1994) | RF |
|
|
|
| Radio and TV performer | (Johnson & Spitz, 1989) |
|
|
||
| Radio announcer | (Demers et al., 1991) | Group 3 |
|
|
|
| Telegraph operator | (Milham, 1985)(Rosenbaum et al., 1994) |
|
|
||
| Telegrapher | (Demers et al., 1991) | Group 3 |
|
|
|
| Communications equipment operator | (Coogan et al., 1996) | Medium |
|
|
|
| Other communications operators | (Loomis et al., 1994b)(Savitz & Loomis, 1995) |
|
|
||
| Electrical and electronic equipment repair (miscellaneous) | (Demers et al., 1991)(Wilkins & Wellage, 1996) | Group 2 |
|
|
|
| Stock handlers and baggers | None |
|
|
||
| Medical technologian | (Davanipour et al., 1997) | Medium |
|
|
|
| Foreman | Telephone company | (Lin et al., 1985) | A (definite) |
|
|
| Chief communications operator | (Loomis et al., 1994b)(Savitz et al., 1998b) |
|
|
||
| 25th percentile of males |
|
||||
| Brickmason | None |
|
|
||
| Shop assistant | Dairy products and bread | (Guénel et al., 1993) | Continuous |
|
|
| Telephone operator | (Loomis et al., 1994b)(Savitz et al., 1998b) |
|
|
||
| Programmer, systems planner | Insurance | (Guénel et al., 1993) | Continuous |
|
|
| Statisticians and scientists | (Coogan et al., 1996) | Medium |
|
|
|
| Social worker | None |
|
|
||
| Employee | Aluminum industry | (Milham, 1985) |
|
|
|
| Military communications worker | (Demers et al., 1991) | Group 3 |
|
|
|
| Air Force pilot | (Davanipour et al., 1997) | Medium / high |
|
|
|
| Blue-collar jobs (non-service) | Aluminum and non-ferrous metal production, smelting and refining | (Rosenbaum et al., 1994) |
|
|
|
| Data entry keyers | (Loomis et al., 1994b)(Sobel et al., 1995) |
|
|
||
| (Sobel et al., 1996)(Savitz et al., 1998b) | |||||
| EDP and card punch operators | (Guénel et al., 1993) | Continuous |
|
|
|
| Electrical and electronics workers | (Spitz & Johnson, 1985) | Narrow definition |
|
|
|
| Electronics workers | (Nasca et al., 1988) | Narrow definition |
|
|
|
| Fork-lift operators | (Wilkins & Wellage, 1996) |
|
|
||
| Heat-treating, equipment, furnace, kiln, and oven operators | (Coogan et al., 1996) | Low |
|
|
|
| Radar operator | (Davanipour et al., 1997) | High |
|
|
|
| Radio operators | (Johnson & Spitz, 1989) |
|
|
||
|
|
|
|
|
|
|
| (Bowman et al., 1988) | Non-office electrical work sites | Spot measurement as close to worker as possible, in direction of most likely field source | 0.07 µT ( microelectronics assemblers);10 µT ( electricians) | Geometric mean | |
| Offices | Spot measurement as close to worker as possible, in direction of most likely field source | 0.31 µT (one secretary with VDT);0.11 µT (3 secretaries w/o VDT) | Geometric mean | ||
| Residences (18) | Spot measurements at several sites; both low & high power conditions | 0.06 µT | Geometric mean | ||
| (Deadman et al., 1988) | 20 workers from 6 electric utilities | Exposure measured over 7-day period for both work and non-work using personal dosimeters | 1.7 µT at work, 0.31 µT for non-work | Geometric mean | Possible misclassification of some work time as non-work |
| 16 workers from 2 office buildings | Exposure measured over 7-day period for both work and non-work using personal dosimeters | 0.16 µT for work, 0.19 µT for non-work | Geometric mean | ||
| (Sahl et al., 1994) | Electricians & substation operators | Exposure obtained for 770 workdays | 2.1 µT (electricians);1.8 (subs. ops.); these were high exposure group | Mean | Occupations studied were classified into 3 groups using fraction of exposures with summary measure > 0.5 µT |
| Office staff, meter readers, & groundmen | 73 workdays of data for 3 classifications of office workers | 0.1, 0.18, 0.23 µT for three classifications of office workers | Mean | Occupations studied were classified into 3 groups using fraction of exposures with summary measure > 0.5 µT | |
| (Barroetavena et al., 1994) | 3 pulp and paper mills (facilities A, B, and C0 | Measurements made at total of 132 locations, and in offices | Facility A - 0.12 µTFacility B - 0.33 µT | Median | Difference in non-office levels attributed tot al electric consumption |
| (Skotte, 1994) | Power frequency MF in elect. util., office, & industrial. workers, & in people living near high power lines | 301 subjects, total of 396 24-hr measurements; 55 subjects were office workers not from utility companies | 0.09 µT (1.8) office workers;0.05 µT (2.1) residences not near high voltage lines | Geometric mean (geometric standard deviation) | |
| (Breysse et al., 1994a) | ELF MF in large payroll department. | Spot measurements | 0.13 to 2.7 µT for office equipment | Range | |
| Personal data for 15 female employees | 0.32 ± 0.15 µT, with range of 0.1-0.65 µT | Mean of personal TWAs, range of personal TWAs | |||
| (Burch et al., 1998) | 194 utility workers | MF & light measured by personal dosimeters 24 h/d for 5 consecutive days; melatonin metabolite excretion measured in urine samples | Distribution workers -- 0.64 ±0.04 at work, 0.5 ±0.04 during sleep;Office and administrative workers -- 0.73 ± 0.03 at work, 0.58 ±0.04 during sleep | RCMS | Standardized rate of change metric (RCMS) for MF was predictor of decreased melatonin excretion |
| (Bracken et al., 1995b) | Utility workers in 13 job classes at 59 sites in 4 countries | 50,000 hrs of MF data taken at 10s intervals by dosimeter; 70% were from work environments | Substation operators 0.7 mT; electricians 0.5 µT
All work categories, while not working 0.09 µT |
Median workday mean
Median non-workday mean |
These two groups had highest exposure by this metric. Utility-specific job classifications had about 1/2 of time-integrated exposure on the job. |
| (Savitz & Loomis, 1995) | 138 905 electric utility workers at 5 US companies | Means in exposure categories 0.12 to 1.27 µT | TWA | No measurements given in Section 2.3 | |
| (Thériault et al., 1994) | 3 cohorts of electric utility workers in Ontario, Quebec, and France | JEM constructed from current occupations and linked to occupational histories | 3.1 µT-years (median)15.7 µT-years (90th percentile) | Cumulative exposure | 90th percentile cut-point for last job was good predictor of 90th percentile cut-point for total job history |
| (Floderus et al., 1996) | Swedish workers | At least 4 personal exposure measurements/occupation for 100 common occupations at 1015 workplaces; used to form JEM | 0.04 µT (earth mover operators); 0.05 µT (concrete workers); 0.19 µT (electrical and electronics engineers and technicians and welders); 0.28 µT (overall); 0.17 µT (median of occupations) | Workday mean |
|
|
|
||||
|
|
|
|
|
|
|
| Denver area (Wertheimer & Leeper, 1982) |
|
|
|
|
|
| Denver area (Savitz et al., 1988) |
|
|
|
|
|
| Seattle area (Severson et al., 1988) |
|
|
|
|
|
| Los Angeles area (London et al., 1991) |
|
|
|
|
|
| Los Angeles area (Preston-Martin et al., 1996b) |
|
|
|
|
|
| Seattle area (Gurney et al., 1996) |
|
|
|
|
|
| Nine states in USA (Linet et al., 1997) |
|
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
| (Wertheimer & Leeper, 1982) Median of spot measurements next to home at point near line |
|
|
|
|
|
| (Savitz et al., 1988) Low power spot measurement in home:Mean |
|
|
|
|
|
| Median |
|
|
|
|
|
| (Severson et al., 1988) Median of a small subsample |
|
|
|
|
|
| (London et al., 1991) Geometric mean of low power spot measurements |
|
|
|
|
|
| Geometric mean of 24-h medians |
|
|
|
|
|
| (Preston-Martin et al., 1996b)Mean of 24-h mean in bedroom |
|
|
|
|
|
| ibid., Mean of 24-h median in bedroom |
|
|
|
|
|
| (Tarone et al., 1998)Mean of 24-h means |
|
|
|
|
|
| Median of 24-h means |
|
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
| (Wertheimer & Leeper, 1982)Outdoor spot measurements > 0.3 µT |
|
|
|
|
|
| (Savitz et al., 1988) Spot measurement > 0.2 µT |
|
|
|
|
|
| (Severson et al., 1988) 24-h mean > 0.2 µT out of a small subsample |
|
|
|
|
|
| (Tarone et al., 1998)24-h mean > 0.2 µT |
|
|
|
|
|
| 24-h mean > 0.3 µT |
|
|
|
|
|